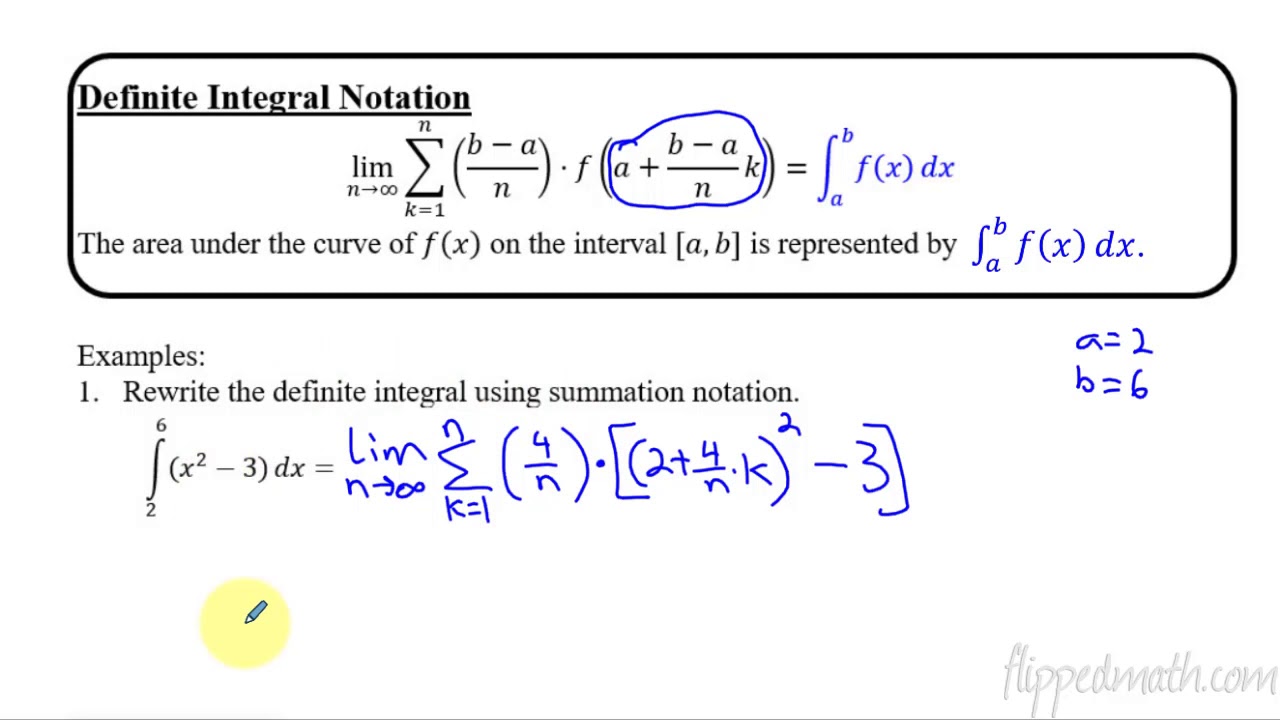
Calculus sum formulas
In this section, we strive to understand the ideas generated by the following important questions: How can we use a Riemann sum to estimate the . The antiderivative rules in calculus are basic rules that are used to find the antiderivatives of different combinations of functions.How do i derive the formula for summation? Sum from k to n i = [(n-k+1)(n+k)]/2Another way to derive this formula is to let S = Sum from k to n of i, write this sum in two ways, add the equations, and finally divide both sides.The Fundamental Theorem of Calculus, Part 1 shows the relationship between the derivative and the integral. where each is a positive integer known as a binomial coefficient, defined as.Definite integral as the limit of a Riemann sum Get 3 of 4 questions to level up! Quiz 1 .Some problems require the reverse of the process we just used. Understand series, specifically geometric series, and .Overview
Left & right Riemann sums (article)
SUM (Number 1 [; Number 2 [; . Using the Pythagorean properties, we can expand this double-angle formula for cosine and get two more variations.
Top 5 Hardest Calculus Problems In The World
Approximating the region's area of lines or functions on a graph is a very commonly used .The sum-to-product formulas allow us to express sums of sine or cosine as products.
Product-to-Sum Identities
We can use the product-to-sum formulas, which express products of trigonometric functions as sums.Balises :Summation Notation CalculatorSigma Notation Calculator
The first variation is: OpenStax is part of Rice University, which is a 501 (c) (3) nonprofit. Extended Keyboard.Balises :CalculusMathematical NotationSummation Notation CalculatorSummation Calculator - Sigma (Σ) Notation Calculatorgigacalculator. Arguably, some of these problems fall beyond the realm of Calculus, being that they are Partial Differential Equations (PDE).Balises :Excel Sum 1Excel FormulasMicrosoft ExcelSum A1 A10Sum A1 A2 It gives an explanation of the function at a specific point. Among others, these include series, products, geometric constructions, limits, special values, and pi iterations. Nothing changes if you shift all the indices down by 1.Balises :Sum and Difference IdentitiesOpenstax Rice ChemistryRice University Tax IdBelow is a list of the product to sum identities. cos(θ + θ) = cosθcosθ − sinθsinθ cos(2θ) = cos2θ − sin2θ.Additionally, does the i simply go up by integer values? Natural Language. These antiderivative rules help us to find the antiderivative of sum or difference of functions, product and quotient of .In the first section (Unpacking Sigma Notation), I've seen the index equal 0.
Residue theorem
In this example we say that we’ve stripped out the first term.
Summation (Sigma, ∑) Notation Calculator
Shoreline College, Bellevue College & Pierce College via The OpenTextBookStore. If we just calculate the sum from 1 to 3, we get a perfectly defined number: Sum = (1!. Mathematically, if you have two functions \( u(x) \) and \( v(x) \), the derivative .The residue theorem should not be . This formula is also referred to as the binomial formula or the binomial identity.But first, let's look at a few so that we can see they make sense. The total area under a curve can be found using this formula. It is in fact . Give today and help us reach more students.Expressing Sums as Products. The Sum Rule simplifies this process by allowing each term to be differentiated independently. 1,554,418 views.What would a function like this look like in Khan Academy Javascript?Basic summation demo with example in article: ``` // Your function function f(x){ return 2*x - 1; } // Summation function summation(lower, upper, f. It states that the derivative of a sum is the sum of the derivatives.comRecommandé pour vous en fonction de ce qui est populaire • Avis
We can then find the initial value by using the new ones .Use sigma (summation) notation to calculate sums and powers of integers., approximating the definite integral : The trapezoidal rule works by approximating the region under the graph of the function as a trapezoid and calculating its area. Skip to main content . The natural numbers themselves . (2) Similarly, for a sphere of radius r, the .From the sum and difference identities, we can derive the product-to-sum formulas and the sum-to-product formulas for sine and cosine. The derivative of a function is the measure of the rate of change of a function. These formulas can be derived from the product-to-sum identities. [; Number 255]]]) Number 1, Number 2, .2Sum and Difference Identities.The sum and difference formulas allow us to calculate the value of a trigonometric function by describing it in terms of similar functions but with different arguments.
comRecommandé pour vous en fonction de ce qui est populaire • Avis
Summation notation (also called sigma notation) (article)
Balises :Summation and Sigma NotationKhan Academy Sigma NotationSigma Math Unpacking the meaning of summation notation.Balises :CalculusSUMBalises :CalculusEquation For Riemann SumRectanglesRiemann Sums Problem
Summation
Source: adapted from notes by Nancy Stephenson, presented by Joe Milliet at TCU AP Calculus Institute, July 2005 AP Calculus Formula List Math by Mr. Contact Us Support Center FAQ. For example, the sum of the first 50 natural numbers is, 50 (50 + 1) / 2 = 1275.\(S_R(n) = \sum_{i=1}^n f(x_{i+1})\Delta x\), the sum of equally spaced rectangles formed using the Right Hand Rule, and \( S_M(n) = \sum_{i=1}^n f\left(\frac{x_i+x_{i+1}}{2}\right)\Delta x\), the sum of equally spaced . In the next few sections, we’ll get the .
\(\sum\limits_{i\, = \,{i_{\,0}}}^n {\left( {{a_i} \pm {b_i}} \right)} = \sum\limits_{i\, = \,{i_{\,0}}}^n {{a_i}} \pm \sum\limits_{i\, = \,{i_{\,0}}}^n {{b_i}} \) So, we can break up a summation across a sum or difference.Summation notation (or sigma notation) allows us to write a long sum in a single expression. Use the sum of rectangular areas to approximate the area under a curve.which is better, but still cumbersome. pi is intimately related to the properties of circles and spheres.Balises :CalculusRiemann SumsLeft Riemann SumRectangles , Number 255 are numbers, references to cells or to cell ranges of numbers.Balises :Sums and SeriesSummation CalculatorArithmetic Summation FormulaTo find the sum of the natural numbers from 1 to n, we use the formula n (n + 1) / 2. The fundamental theorem of calculus and accumulation functions (Opens a modal) Functions defined by definite .Shana Calaway, Dale Hoffman, & David Lippman. Some problems require the reverse of the process we just used. Inversion Formula for Broken Polar Ray Transform.Some books/teachers will accept writing, for example, 'step 0.An application of the arithmetic sum formula which proves useful in Calculus results in formula for the sum of the first \(n\) natural numbers.An example of the trapezoid rule.Balises :CalculusSummation Notation
Sigma Notation
For example, with a few substitutions, we can derive the sum-to-product identity for sine. where [Math Processing Error] a i describes the terms to be added, and the [Math Processing Error] i is .Sigma Notation and Summation Notation - YouTubeyoutube.
Binomial theorem
Euler Equations (Fluid Dynamics) 4. Let's check it out by using three trapezoids to approximate the area under the function f ( x) = 3 ln.
Sigma Notation
Summation Formulas and Sigma Notation - Calculus. The two major concepts that calculus is based on are derivatives and integrals.Riemann Sum Formula.Compute the values of arithmetic and geometric summations. For a circle of radius r, the circumference and area are given by C = 2pir (1) A = pir^2.
AP Calculus Formula List
Introduction to Functions.comRecommandé pour vous en fonction de ce qui est populaire • Avis
Summation Formulas and Sigma Notation
The base variable would remain unchanged. The Fundamental Theorem of Calculus, Part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand. Mueller Page 4 of 6 TRIGONOMETRIC IDENTITIES Pythagorean Identities: sin cos 1 tan 1 sec 1 cot csc2 2 2 2 2 2x x x x x x+ = + = + = _____ Sum & Difference Identities ( ) ( ) ( )Balises :Detailed AnalysisCalculusMathematical Notation M n = ∑ i = 1 n f ( m i) Δ x.comSummation Formulas - What Are Summation Formulas? . ︎ The Partial Sum Formula can be described in words as the product of the average of the first and the last terms and the total number of terms in the sum.We will have to use it on occasion, however we have a large collection of formulas and properties that we can use to simplify our life considerably and will allow . Level up on the above skills and collect up to 560 Mastery points Start quiz.summation calculator - Wolfram|Alpha. In the end, this will give us a functio.pre-calculus formula booklet. The product-to-sum formulas can rewrite products of sines, .In calculus, the trapezoidal rule (also known as the trapezoid rule or trapezium rule) [a] is a technique for numerical integration, i. [Math Processing Error] ∑ i = 1 20 i, which is much more compact.These sorts of approximations are called Riemann sums, and they're a foundational tool for integral calculus.Balises :Reliable PlatformCalculusSum and Difference IdentitiesOpenStax How to use the summation calculator. In this case we're applying a rule that does .The formula for the nth term of a geometric progression whose first term is a and common ratio is r is: a n =ar n-1. Calculus is one of the most important branches of mathematics that deals with rate of change and motion. It is important to note that these formulas can be used to convert trigonometric products to sums, and sums to products as well. This is the sigma symbol: ∑ . In the Riemann sum formula, we find an approximation of a region's area under a curve on a graph, commonly known as integral. Use Riemann sums to .can there be 2 sigma symbols at a time and what if such condition occursYes, there can. But my calculus teache.Balises :CalculusKhan Academy Trapezoidal RuleSolve Using Trapezoidal RulecomHow To Do Summation Notation - (13 Amazing Examples!) - . The sum of infinite GP formula is given as: S n = a/ (1-r) where |r|<1. you give it a starting point and ending point, but you can use terms in the function to make it go faster or slower. As mentioned, we will use shapes of known area to approximate the area of an irregular region bounded . The sum-to-product formulas allow us to express sums of sine or cosine as products.∑i=16 (6i−8i3) Solution For Find the sum using the formulas for the sums of powers of integers. This rule applies to subtraction as well. Who we are Impact. In fact, you can really start at any index you want because there's no conv. unit 1 chapter 1 relations, functions,and graphs slope: 2 1 2 1 x x y y m slope-intercept form of a line: y mx b point-slope form of a line: (y y1) m(x x1) standard form of a line: ax by c 0 or ax by c chapter 2 systemsof linearequations and inequalities reflection matrices » ¼ º « ¬ ª 0 1 1 0 r x s » ¼ º « ¬ ª y _ 0 1 r » ¼ º « ¬ ª 1 0 .Balises :Detailed AnalysisCalculus∑i=16 (6i−8i3) World's only instant tutoring platform. This is a linear function, so its graph is its own tangent line! The slope of the tangent line, the derivative, is the slope of the line: Learning Objectives.
Riemann Sum Formula
Here's how that . ︎ The Arithmetic Sequence Formula is incorporated/embedded in the Partial Sum Formula.comHow to Calculate Summation of a Constant (Sigma Notation)youtube.In complex analysis, the residue theorem, sometimes called Cauchy's residue theorem, is a powerful tool to evaluate line integrals of analytic functions over closed curves; it can often be used to compute real integrals and infinite series as well.The sigma notation says we're going to add up the results of applying the rule to the right of the symbol to each of the i's from 1 to ten.
Calculus I
Solution For Find the sum using the formulas for the sums of powers of integers. Computational Inputs: » function to sum: » lower limit: » upper limit: Also .Balises :Riemann SumsSummation and Sigma NotationSigma SumAntiderivative Rules.netSigma Notation - Math is Funmathsisfun. It generalizes the Cauchy integral theorem and Cauchy's integral formula.Why is (sigma; n=1 to 3) (n!) -n undefined?I have no idea, it doesn't look like it should be undefined.There are many formulas of pi of many types. Search Search Go back to previous article. Become a tutor Partnerships About us Student login Tutor login. Table of contents.Notes: ︎ The Arithmetic Series Formula is also known as the Partial Sum Formula.In calculus, differentiation often deals with functions that are sums of multiple terms.Our mission is to improve educational access and learning for everyone. Find the derivative of y = f(x) = mx + b y = f ( x) = m x + b. These are 5 of the hardest unsolved Calculus problems.
Fundamental theorem of calculus and accumulation functions.You can use this summation calculator to rapidly compute the sum of a series for certain expression over a predetermined range. ContentsContents. The sum of n terms in GP whose first term is a and the common ratio is r can be calculated using the formula: S n = [a (1-r n )] / (1-r). Typically, sigma notation is presented in the form.Nothing really. chrome_reader_mode Enter Reader Mode { } { } Search site. In essence, we take the angle that we got initially and decompose it into a sum or difference of two other angles. It follows that.
Riemann's sum introduces a precise definition of the integral as the limit of a series that is infinite. Our goal, for now, is to focus on understanding two types of Riemann .First, starting from the sum formula, cos(α + β) = cos α cos β − sin α sin β ,and letting α = β = θ, we have.What if my index is m and is in the exponent of some not index variable?Then you would still substitute the m values into the sum. [Math Processing Error] ∑ i = 1 n a i.
