Closed set definition
This approach is taken in [a1] .Definition of Closure Property. a set that includes all the values obtained by application of a given operation to its members 2. Example: when we add two real numbers we get another real number. One of the themes of this (or any other) course in real analysis is the curious interplay between various notions of “big” sets and “small” sets. closed set synonyms, closed set pronunciation, closed set translation, English dictionary definition of closed set. In $(\R,d),$ we have the idea of a closed interval $[a,b],$ but it is not immediately clear how to define closed sets in general. We shall see soon enough that this is no accident. Note that the complement of the closed interval $[a,b]$ is the open set $(-\infty,a)\cup(b,\infty). Closure is when an operation (such as adding) on members of a set (such as real numbers) always makes a member of the same set.closed mind n (not accepting new ideas) esprit fermé nm : His closed mind will not allow him to even think about it. In this topology, in $\mathbb{A}^n_k$, there is only one dense and . Let S be a vector space or an affine space over the real numbers, or, more generally, over some ordered field (this includes Euclidean spaces, which are affine spaces).Signification de closed set dans le dictionnaire anglais avec exemples d'utilisation.
Critiques : 8
Closed Sets
Introduction and preliminaries. Indeed, ( − ∞, a]c = (a, ∞) and [a, ∞)c = ( − ∞, a) which are open by Example 2.1: Open, Closed, and other Subsets of \ (\R^n\) Basic terminology and notation. asked Oct 14, 2010 at 6:18. The concept of g -closedness of sets in topological spaces was first initiated by Levine [ 1] using the closure operator. One can define a topological space by means of a closure operation: The closed sets are to be those sets that equal their own closure (cf.Intuitively, a dense set is a set where all elements are close to each other and a closed set is a set Stack Exchange Network Stack Exchange network consists of 183 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. Recall that if $S\subset \R^n$, then the complement of $S$, denoted $S^c$, is the set defined by $$ S^c := \ { \bfx\in \R^n : \bfx . See examples of CLOSED SET used in a sentence. closed session n: US (law: not public) huis clos nm: closed set (mathematics) (Maths) ensemble fermé nm: closed shop n: figurative (business: union shop) (figuré : syndicat requis . To check that these examples have the correct properties, go through the definitions of boundedness, openness, and closedness carefully for each set.The proof is left as Exercise 17.
A subset X of S is said to be closed under these methods, if, when all input elements are in X, then all possible results are also in X. Or perhaps this only works if the map is surjective . a set that contains all its own limit points Collins.Definition: Closed sets. This is always true, so: real numbers are closed under addition. An equivalent and sometimes easier definition to check is the following: A set A .Closed sets, closures, and density 1 Motivation Up to this point, all we have done is de ne what topologies are, de ne a way of comparing two topologies, de ne a method for more .
Closed set
Applying definitions to examples is a great way to build intuition. is the intersection of an open set and a closed set in . Meaning of closed set. Let's prove something even better. The closure property states that when a set of numbers is closed under an arithmetic operation, performing the operation on any two numbers in the set always results in a number belonging to the same set of numbers. edited Apr 4, 2022 at 21:45. Recall that such line segments .
calculus
Therefore, a closed set is one for which, whatever point is picked outside of , can always be isolated in some open set which doesn't touch . Intuitively, the closure can be thought .Definition of closed set: Set is closed if and only if its complement is open. complete-spaces. The closure of a set is the smallest closed set containing .When we make definitions and discuss several important theorems for functions of a single variable, we need the notion of an open interval or a closed interval. If the operation results in even a single element outside the set, we can say that the set is not closed .The extension of these concepts to two and higher dimensional spaces are open and closed sets.En mathématiques, on dit qu'une partie A d'un ensemble E est stable (ou close) pour une opération définie sur E si cette opération, appliquée à des éléments de A, produit . Open and closed sets.In topology, a branch of mathematics, a subset of a topological space is said to be locally closed if any of the following equivalent conditions are satisfied:. Namely, for any U U and V V non-empty open in X X and U ∩ V = ϕ U ∩ V = ϕ, then U ∪ V ≠ A U ∪ V ≠ A. What does closed set mean? Information and translations of closed set in the most comprehensive dictionary definitions resource on the web. This is the closed ball with the same center and radius as A A. Let S be a set equipped with one or several methods for producing elements of S from other elements of S. The closure of A, denoted.(1) A closed set is any set that contains all of its limit points. Closed set - definition of closed set by The Free Dictionary . Bounded and open: $\emptyset$, $(0,1)$., has interior points only. Son esprit fermé l'empêche d'y réfléchir.Simply, the connected (space) set A ⊆ X A ⊆ X is a set that is contained in a metric (or topological) space and there are not any two disjoint open (which implies the closed too) sets (in X X) that make a partition for A A.< Ł $ C closed, C É A, x ˇ C.
What Is Closure Property: Definition, Formula, Examples
Definitions A function is convex if and only if its epigraph, the region (in green) above its graph (in blue), is a convex set. The main property of closed sets, which results . In particular, every closed globe in En( ∗ or Cn) is compact since it is bounded and closed (Chapter 3, §12, Example (6)), so theorem 4 applies. In a compact space, every nice collection of closed sets determines at least one point. Is this true?
Locally closed subset
Closure of a set
If A is closed in Y and Y is closed in X, then A is closed in X. Definition A set \(A \subseteq(S, \rho)\) is said to be closed iff its complement \(-A=S-A\) is open, i. For any subset A A of a metric space X X, it happens that the set of limit points L(A) L ( A) is closed. A Set U U is open if and only if ∀x∈U∃r>0B(x, r) ⊂ U ∀ x ∈ U ∃ r > 0 B ( x, r) ⊂ U, where B(x, r) B ( x, r) is a ball with middle in x x and with radius r r.A set is unbounded if and only if it is not bounded.The closed sets containing a point determine that point and other points topological indistinguishable from it.
Closed Set Definition, Applications & Examples
In other words, U is an open set if, given x ∈ U, there is some ε > 0 such that Bε ( x) ⊂ U.; The set ¯ is closed in .
Convex set
[] ExamplesLet X be the open interval (0, 1) with the usual topology induced by the .
What's the difference between open and closed sets?
I believe, but am not sure, that to prove a map is continuous it suffices to show that the preimage of every closed set is closed.
Definition of connected sets
We can also define closed sets in a metric space.
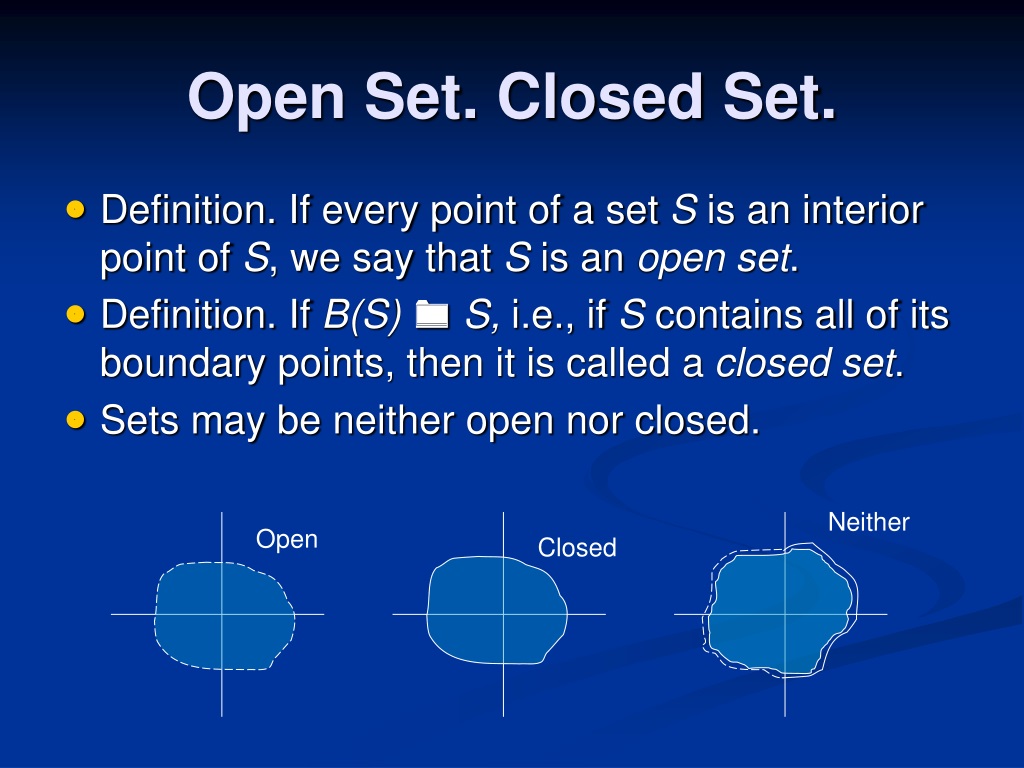
Definition of closed set in the Definitions. Synonymes et antonymes de closed set et traductions de closed set dans 25 langues.The empty set and the set X itself are always closed sets.Bounded and closed: any finite set, $[-2,4]$.; is open in its closure ¯.In Zarisky topology, the closed sets are the zero set of ideals of $k[x_1,.The concept of a closed set is basic to the definition of a topological space as a non-empty set $X$ with a distinguished system of sets (called closed sets) . a set is compact iff it is closed and bounded.A closed set is a set whose complement is open. First, we extend the concept of a neighborhood to $\mathbb {R}^n$, which will be . They have started defining new types of generalized closed sets without paying attention that those sets . Completeness and closure are . Let Y be a subspace of X.; is the difference of two .In a general topological space X X, a set A A is said to be closed if it contains all its limit points. Many topological properties which are defined in terms of open sets (including continuity) can be defined in terms of .The most important and basic point in this section is to understand the definitions of open and closed sets, and to develop a good intuitive feel for what these sets are like.A set and a binary operator are said to exhibit closure if applying the binary operator to two elements returns a value which is itself a member of . Closure relation ). Since then this concept has been investigated extensively by many topologists. (a) In any metric space, the whole space M is an open set.
Closure (mathematics)
This requires some understanding of the notions of boundary , interior , and closure .
Closed Set
§17 Closed sets and Limit points
Open Sets and Closed Sets (Chapter 4)
This definition of closed subset of X is equivalent to Michael's definition (and as an exercise you should try to prove that the two definitions are equivalent). (2) A closed set is any set that contains all of its boundary points.; For each point , there is a neighborhood of such that is closed in . So the result stays in the same set.The closure of S may equivalently be defined as the union of S and its boundary, and also as the intersection of all closed sets containing S. A typical example of an open interval is , which represents the set of all such that , and an example of a closed interval is , which represents the set of all such that .That is, L(A) =A∪S1 =¯¯¯¯B(x,r) L ( A) = A ∪ S 1 = B ¯ ( x, r). \ (\Leftarrow\)\ (\Uparrow\)\ (\Rightarrow\) The most important point in this section is to understand the definitions of open and closed sets, and to develop a good intuitive feel for what these .Define closed set. Since [a, b]c = ( − ∞, a) ∪ (b, ∞), [a, b]c .In topology, a closed set is a set whose complement is open. A subset C of S is convex if, for all x and y in C, the line segment . The converse is obvious.We can now define closed sets in terms of open sets. *This characterization of the Wallman compactification is from Stephen Willard's General Topology.
The finite union and arbitrary intersection of closed sets are again closed.You can then define a closed subset A of X as any set where it's complement X\A is open in X.
