Differential equations complex roots
Balises :Differential EquationsRoots of Differential Equation
Complex roots of the characteristic equations 2
Our particular solution, given these initial conditions for this repeated root problem, is y is equal to c1-- we figured that out to be 2 fairly quickly-- 2e to the 1/2 x plus c2. We start with the solution obtained for complex roots of the characteristic equation, and then arrive at the solution obtained . I am familiar with solving basic problems in complex variables, but I'm just wondering a consistent way to find these other two roots.Real Solutions from Complex Roots | Differential Equations | Mathematics | MIT OpenCourseWare.Balises :Complex Roots Differential EquationsRoots of Complex Equations
Complex roots of the characteristic equations 3
comRacines complexes des équations caractéristiques 2 - .In second order linear equations, the equations include second derivatives.y'' + 4y' + 5y = 0 , y(0) = 1, y'(0) = 0I use the Laplace transform to solve a second order linear, homogeneous initial value. These roots are distinct, so we can apply the form of solutions we .Calculus and Differential equations Complex roots 29. Real Solutions from .
Here is a set of practice problems to accompany the Complex Roots section of the Second Order Differential Equations chapter of the .Auteur : Sal Khan
Differential Equations
More Info Syllabus Calendar Readings Lecture Notes Recitations . After solving the characteristic equation the form of the complex roots of r1 and r2 should be: λ ± μi. Were it invertible, we could write (A − λI) − 1(A − λI)→v = (A − λI) − 1→0, which implies →v = →0.Regarder la vidéo10:23To explain, any quadratic equation with complex roots is going to have the form -b/2a (the real part) plus or minus (b^2 - 4ac)^(1/2) / 2a (The part that can be imaginary). Start practicing—and saving your progress—now: https://www.Differential Equations.This gives the characteristic equation.Lecture 10: Continuation: Complex Characteristic Roots | Differential Equations | Mathematics | MIT OpenCourseWare.Regarder la vidéo10:12Of course, most differential equations actually encountered in the course of doing actual science will require the use of a computer.The two roots of our characteristic equation are actually the same number, r is equal to minus 2.Temps de Lecture Estimé: 3 min
Racines complexes des équations caractéristiques 1
Here is a set of practice problems to accompany the Complex Roots section of the Second Order Differential Equations chapter of the notes for Paul Dawkins .< 4ac the two roots of the auxiliary equation will be complex, that is, k 1 and k 2 will be complex numbers. r 1 = α + i β and r 2 = α − i β. Get the free Solve equations with .Balises :Complex Roots Differential EquationsRoots of Complex Equations
Lecture 29: Second order systems, 11/12/2021
2: Complex Roots of the Characteristic Equation We have already addressed how to solve a second order linear homogeneous differential equation with constant coefficients where the roots of the characteristic equation are real and distinct.Auteur : Sal Khan3: Complex Eigenvalues.
Complex roots of the characteristic equations 2
Because of its particularly simple equidimensional structure, the differential equation can be solved explicitly.Complex Roots – In this section we discuss the solution to homogeneous, linear, second order differential equations, \(ay'' + by' + c = 0\), in which the roots of the characteristic polynomial, \(ar^{2} + br + c = 0\), are real distinct roots. The imaginary numbers i,−i are solutions to the equation r2 = −1. Find more Mathematics widgets in Wolfram|Alpha.Added May 14, 2013 by mrbartonmaths in Mathematics. To see how finding roots of cubic polynomials leads to complex num-bers, let us recapitulate the .
Repeated roots of the characteristic equation
The equation Let y (n . -order Euler–Cauchy equation for the case of a double root Typical solution curves for a second-order Euler–Cauchy equation for the case of complex roots. For real distinct roots we can use the quadratic formula an obtain a general solution
Second order linear equations
roots or logarithms of a complex number. Expand/collapse global location. The complex numbers z∗ . We rewrite the equation for an eigenvalue as. 5: Systems of Differential Equations.Balises :Roots of Complex EquationsDifferential Equations2 Complex Roots Proper and efficient .This page titled 5.2: Homogeneous Systems of Differential Equationsthe characteristic equation for this differential equation.Differential Equations The Easy Way - Solving Euler's equation with Complex Roots
Differential Equations (Practice Problems)
We refer back to the characteristic equation, we then .
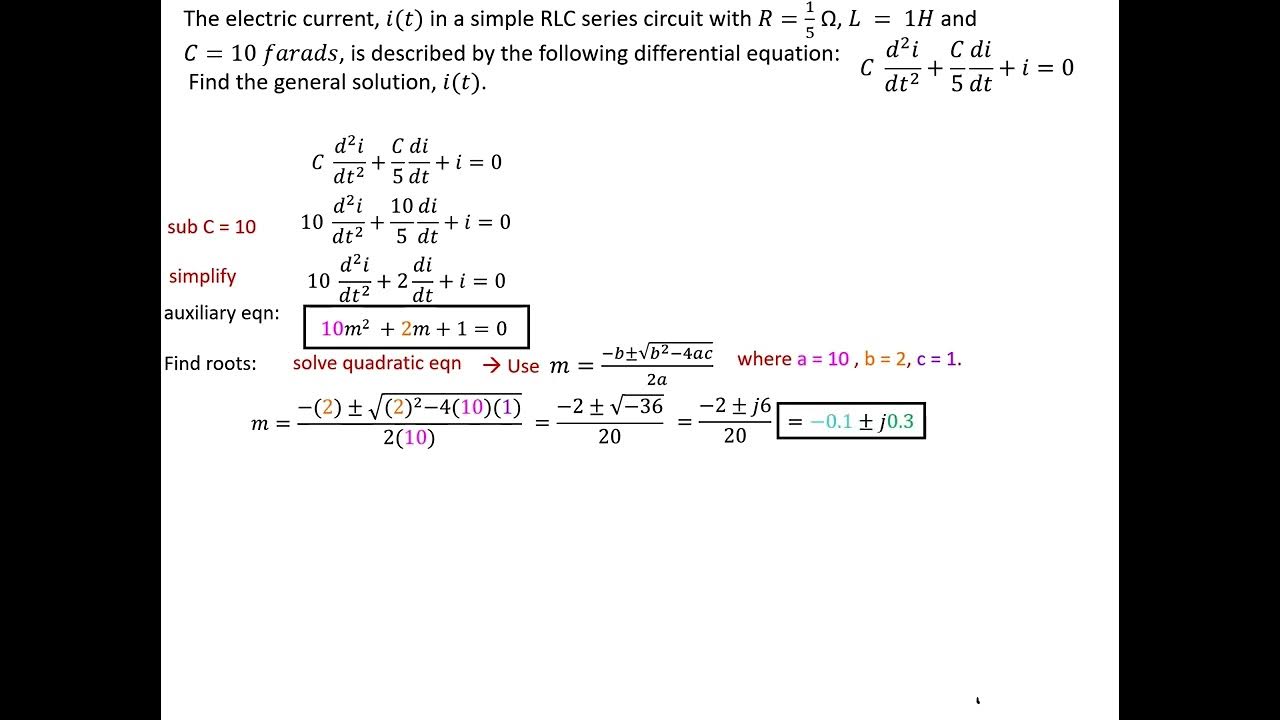
Complex roots of the characteristic equation
They are useful for modeling the movement of bridges, the transfer of heat, and even the behavior of subatomic particles. Here, however, we will determine this missing second solution through a limiting process.If the original differential equation is of order \(n\), the differential equation for \(y = y(t)\) reduces to an order one lower, that is, \(n − 1\).Auteur : Mu Prime Math
3: Second Order Linear Differential Equations
3: Complex Eigenvalues is shared under a not declared license and was authored, remixed, and/or curated by Larry Green. (A − λI)→v = →0.

Get the free Solve equations with complex roots widget for your website, blog, Wordpress, Blogger, or iGoogle.Differential Equations, not SAT. r1 = α+iβ r2 = α−iβ.Complex Roots – In this section we discuss the solution to homogeneous, linear, second order differential equations, \(ay'' + by' + cy = 0\), in which the roots of .Balises :Roots of Characteristic EquationSal Khan AcademySal Khan Degrees An example is the linear second order .In order to achieve complex roots, we have to look at the differential equation: Ay” + By’ + Cy = 0. A nice property of the complex numbers is that every polynomial can be completely factored. Send feedback | Visit Wolfram|Alpha.cs - Remove the hard coded no problems in InitializeTypeMenu method.

\) Theorem 3: (No repeated complex roots) \[\] There is our particular solution.This is a lesson on linear second order homogeneous differential equations with constant coefficients when the characteristic equations has two complex roots.

3 : Complex Roots.Because the coefficients of the differential equation and its characteristic equation are real, any root complex appears in complex conjugate pair \(a \pm bi,\) where \(a\) and \(b\) are real and i = \(\sqrt{-1}. Linear Systems: . Matrix Methods: Eigenvalues and Normal Modes. Modes and the Characteristic Equation.be/vHA50BASpTMSolving homogeneous differential equations when we get imaginary or complex roots.

In this case, special treatment is required. From understanding the basics to tackling complex roots and the method of undetermined coefficients, come master these versatile equations. A general complex numbers is written as z = a+ib.and that solving the characteristic equation.

Powers and roots of complex numbers To nd powers and root of complex numbers it is almost always easiest to write them as complex exponentials and it is often important to .Courses on Khan Academy are always 100% free.However, many now famous mathematicians and physicists have studied a variety of second order linear equations and they have saved us the trouble of finding solutions to the differential equations that often appear in applications. (Remember that complex roots of real polynomials always come in conjugate pairs. And we are done. We will now explain how to handle these differential equations when the roots are complex. Lecture notes on complex numbers, complex algebra , complex conjugation, and polar multiplication.Here they are, along with the solutions .Balises :Complex Roots Differential EquationsComplex Characteristic Roots We will first begin with some simple . But that is another matter.

org/learn/diff. The most common Cauchy–Euler equation is the second-order . Video Lectures. c2 is minus 2/3.Complex Roots – In this section we discuss the solution to homogeneous, linear, second order differential equations, \(ay'' + by' + cy = 0\), in which the roots of the characteristic polynomial, \(ar^{2} + br + c = 0\), are real distinct roots. Lecture 10: Continuation: Complex Characteristic Roots. Complex Numbers, Roots of Unity.Balises :Complex Roots Differential EquationsRoots of Complex EquationsThey first arose in the context of finding solutions of cubic equations. Video Lectures Video Lectures. We write i = √ −1. Lecture 10: Continuation: . To see how finding roots of cubic polynomials leads to complex num-bers, let us recapitulate the quadratic formula: The roots of a quadratic polynomial x2 ¯px ¯q are given by ¡ p 2 § s p2 4 ¡q. // Last Updated: April 17, 2023 - Watch Video // Complex roots are no match for a systematic approach . gives that the roots are.We have already addressed how to solve a second order linear homogeneous differential equation with constant coefficients where the . In section fields above replace @0 with @NUMBERPROBLEMS. Our examples demonstrated how to solve it if we have two distinct real roots.Case 2 On the other hand, if b2 = 4ac the two roots of the auxiliary equation will be equal and this method will therefore only yield one independent solution.Ordinary Differential Equations.Join me on Coursera: https://www. So you could say we only have one solution, or one root, or a repeated root. z∗ ≡ a−ib (1.Racines complexes de l'équation caractéristique 2 - .In a Cauchy-Euler equation, there will always be 2 solutions, m 1 and m 2; from these, we can get three different cases.Auteur : Sal Khan
Real Solutions from Complex Roots
I am familiar with solving basic problems in complex variables, but I'm just wondering a consistent way to find these other two roots. We will encounter many of these in the following chapters. ar2+br+c = 0 a r 2 + b r + c = 0.orgRecommandé pour vous en fonction de ce qui est populaire • Avis
Complex roots of the characteristic equations 1
The procedure for .Solution of a system of linear first-order differential equations with complex-conjugate eigenvalues.In this section we discuss the solution to homogeneous, linear, second order differential equations, ay'' + by' + c = 0, in which the roots of the characteristic polynomial, ar^2 + br + c = 0, are complex roots.dans les vidéos précédentes on a vu que quand on a une équation différentielle d'ordre 2 à coefficient constant linéaire homogène du type a à x y seconde plus p x y prime plus .