Epsilon delta problem examples
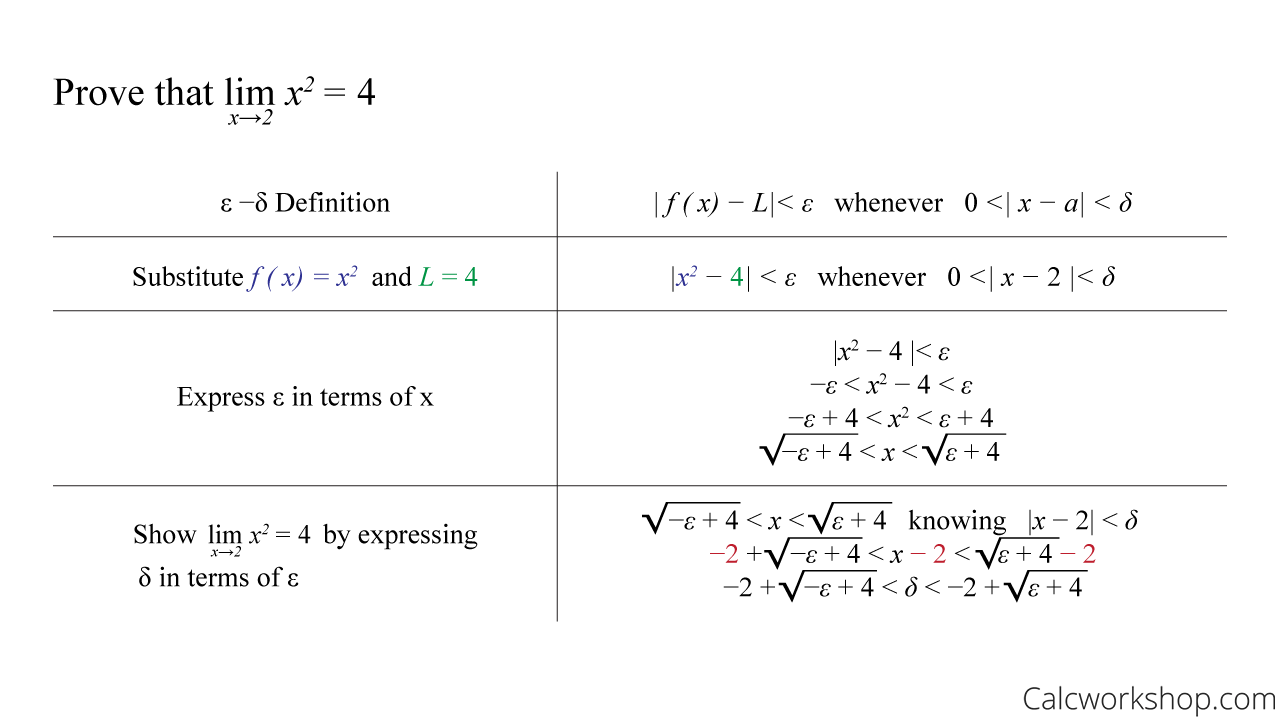
Hence, for all , is the number fulfilling the claim. 0 <|x −x0| < δ |f(x) − f(x0)| 0 ∃ δ > 0 s. 1+x2 whenever 0 < |x − 2| 0.52K subscribers. lim x→−∞ 1 x =0 lim x → − ∞. First note that |2a| is always . Prove that lim x→a x 2 = a 2. The Math Tutor. (Since we leave a arbitrary, this is the same as showing x 2 is continuous.Finally, we have the formal definition of the limit with the notation seen in the previous section. As we know the epsilon-delta definition of continuity is: For given.Watch the next lesson: https://www. September 14, 2010. 4, we just have .Epsilon-Delta Proof. Let’s show this is true by this de nition. Tobias Schottdorf.
Calculus I
if 0 < |x - a| < δ then |f(x) - L| < ε . By saying that for any δ > 0 and any ε > 0 then: 0 <|z − i| < δ . An example is the following proof that every linear function () is continuous at every point .An example is the following proof that every linear function f (x)=ax+b (a,b in R,a!=0) is continuous at every point x_0.Example 1, usingε-δdefinition, that lim x→ 4 (x+ 11) = 15.Balises :Epsilon-Delta Definition of LimitFinding Epsilon and Delta LimitsBalises :Epsilon-Delta Definition of LimitCalculus
Further Examples of Epsilon-Delta Proof
That is, the definition says that f is continuous at a if for each ϵ > 0, there exists δ > 0 such that if | x − a | < δ, then | f(x) − f(a) | 0.
The Epsilon-Delta Definition of a Limit
To do this, we modify the epsilon-delta definition of a limit to give formal epsilon-delta definitions for limits from the right and left at a point.
Continuity and uniform continuity with epsilon and delta
Choose δ = ϵ .Describe the epsilon-delta definition of a limit. We must prove that. Stack Exchange Network.Taille du fichier : 148KB
Exercises
We find |3x − 1 − 5| < 1 ⇐⇒ |3(x − 2)| < 1 ⇐⇒ |x − 2| < 1/3. limx→3(4x − 1) = 11 lim x → 3 ( 4 x − 1) = 11. That is, prove that if lim x→a f(x) = L and lim x→a f(x) = M, then L = M.
Proving the limit of a quadratic — Mathwizurd
These are the practice problems for Epsilon-Delta definition (precise definition).< |x − a| < δ implies |x 2 − a 2 | < ε. Conceptually, your task in such a proof is to step into Player’s shoes: given that Hater can throw any >0 at you, you need to nd a scheme for turning that into a >0.I'm trying to understand the proof of the following limit using epsilon-delta definition. So you can tell .Limits With Epsilon-delta definition! (9 examples) | Practice Problems | Calculus I - YouTube. The limit of f(x), as x approaches c, is L, denoted by.Some examples should make this clear.Balises :CalculusEpsilon Delta Limit Practice ProblemsDelta Epsilon Limit Examples
Formal definition of limits Part 3: the definition
This probably still makes your head hurt/spin and it probably won’t stop.
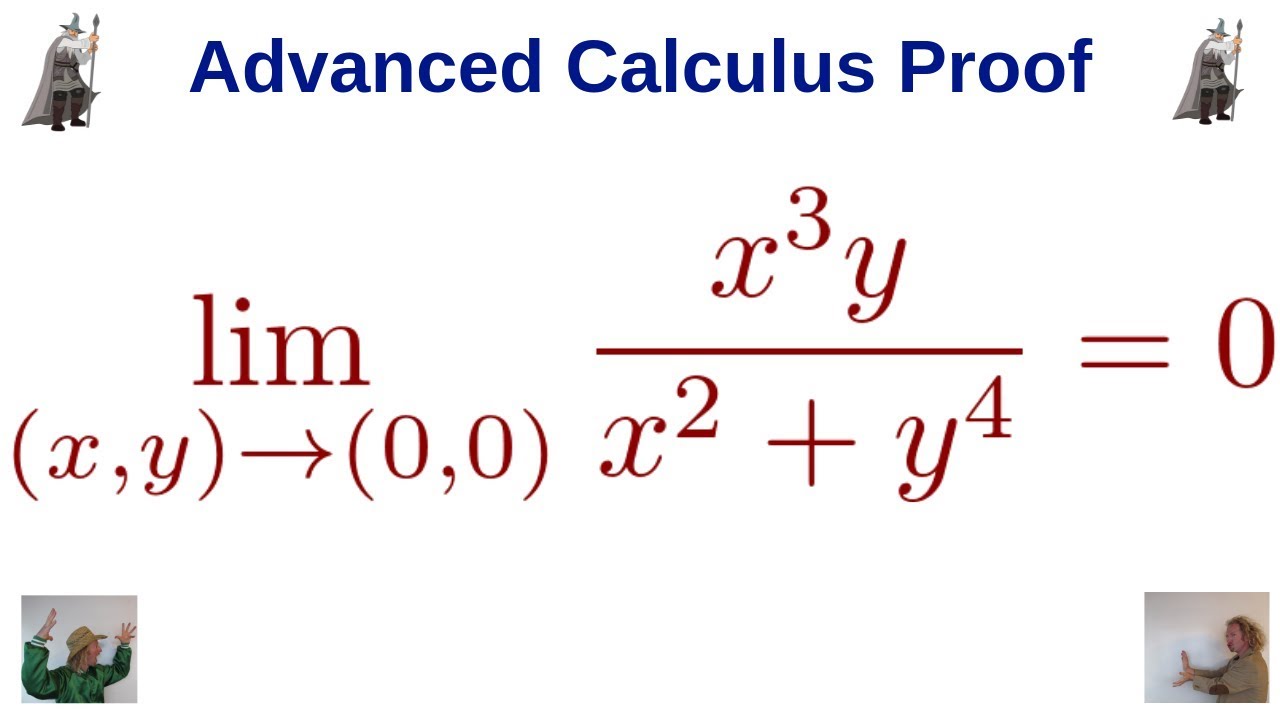
Proving continuity in multivariable calculus using $\epsilon
It suffices to show that there exists a δ > 0 such that for every y in the domain The expression 4x − 1 4 x − 1 in the last example was a linear one, and led to a δ δ that could be used in the definition which was really a very simple .Struggling with calculus limits? This ultimate study guide covers all the essential topics from Calculus 1 and Calculus 2 you need to master!
Balises :Epsilon-Delta Definition of LimitCalculusLimits
Epsilon-Delta Proof
Balises :Epsilon Delta ProofLimit Proof ExamplesFile Size:68KBPage Count:4
Limits With Epsilon-delta definition!
Here’s a quick example of one of these limits. Are the steps right? For example . Solution: Given >0,letδ= /3. Try to do as many problems as you can before looking at the solutions. We wish to work backwards to gure out how close to (0;0) the point (x;y) must be to ensure that this inequality is true.The textbook im using only has stated the definition of limit with examples explained intuitively and without full rigour, so im pretty lost on multivariable limit/continuity problems.Balises :CalculusDefinition of Continuity of A FunctionEpsilon-Delta Proof of ContinuityProve that the limit as x goes to 2 of (x^2+x-6)/(x-2) using the. x= 4 We must rst determine what aand Lare. Say we’re looking at f(x) = 3x − 1.org/math/differential-calculus/limits_topic/epsilon_de. We see that we require | 5r3cos3(θ) − r4cos2(θ)sin2(θ) | < ϵ.) Proof: Let ε > 0. Stack Exchange network consists of 183 Q&A communities including Stack Overflow, the largest, most .< 1 and y < 2 respectively, and note the inequality are strict, since this interval is not closed.$\begingroup$ I think any function that’s not linear requires a bit of ingenuity when dealing with the inequality (I think the use of “min” is a tricky thing, personally, and I can’t think of a nonlinear example that doesn’t require it.What it means for the limit to exist is that you will always be able to find a range of inputs around our limiting input, some distance delta away from 0 0, so that any input within a distance delta of 0 0 corresponds to an output with a distance epsilon of 12 12. That’s not particularly useful, for determining what the prediction will be but it’s better than the $[-10^{100}, 10^100]$, so will help a lot for . However, let’d make more sense of this with some examples. Prove that the limit as x goes to -4 of (x^2-1) = 15 using the. We claim that the choice δ = min ε |2a| + 1 , 1 is an appropriate choice of δ. As such, we can definitively say as a consequence of the epsilon-delta definition of a limit that.So these bounds tell us that for a perturbation delta of $\epsilon=0.is to say that f(x) can be made as close to L as desired, by making x close enough, but not equal, to p.As in most ϵ − δ proofs, we start at the inequality we want to be true, then work backwards to find the necessary restrictions on δ. epsilon, delta definition of a limit.

Epsilon-Delta Proof
is continuous at. Skip to main content. Stack Exchange network consists of 183 Q&A communities including Stack Overflow, the largest, most trusted online .For example, in your example, the interval for (x,y) is (1,2). somewhere in the range $[-2998, 2305]$. We have to think about what it means to approach a particular value.An epsilon-delta definition is a mathematical definition in which a statement on a real function of one variable having, for example, the form for all neighborhoods of there is a neighborhood of such that, whenever , then is rephrased as for all there is such that, whenever , then . These kind of problems ask you to show1 that lim x!a f(x) = L for some particular fand .To make an example, how would you show that is continuous? | √x − √a | = | x − a √x + √a | < | δ √x + √a | < | δ √a | = ε for δ = ε√a. Definition 1: The Limit of a Function f.Explore the epsilon-delta definition of limits, which states that the limit of f (x) at x=c equals L if, for any ε> Note that f(x) =x+ 11, and that we are trying to show that the limit of f, as x−→4, isL= 15. In this case, a= 4 (the value the variable is approaching), and L= 4 (the .The basic idea of an epsilon-delta proof is that for every y-window around the limit you set, called epsilon ($\epsilon$), there exists an x-window around the point, . The precise meaning of limx → af(x) = L states that for every number ε > 0, there is a number δ > 0 such that.1 Numerical Example. This is probably the first place most students ever manipulate . The precise meaning of limx → af(x) = L states that for every number . They key point is that this is true for any epsilon, no matter how small, you .Example of a simple epsilon-delta proof.

Just as we first gained an intuitive understanding of limits and then moved on to a more rigorous definition of a limit, we now revisit one-sided limits.
Epsilon-delta exercises

Lis our limit if for a chosen epsilon, delta exists where my interval on the x-axis gives values of x where f(x) is within epsilon of L. limz→i iz3 − 1 z + i = 0. Problem: Givean -δproofthatlim x→2(3x−1) = 5.How to use the epsilon-delta definition of the limit to prove the limit of a function f as x approaches a is L . Prove: f(x) = x.
Solving epsilon-delta problems
There will probably be at least one epsilon-delta problem on the midterm and the nal. The epsilon-delta definition . ε > 0 ∃δ > 0 s.4 Example: a \delta-epsilon proof The kind of problem commonly called a \delta-epsilon proof is of the form: show, using the formal de nition of a limit, that lim x!cf(x) = Lfor . Example: Proving a Statement about the .
The Epsilon-Delta Limit Definition: A Few Examples
The resulting bound on distance, , will depend on .Balises :Proving Limits with EpsilonEpsilon Delta Proof SolverMinimum in Quadratics lim x!3 4x 5 = 7 lim x → cf(x) = L,

Functions of a single variable ε, δ)-definition of limit For the depicted f, a, and b, we can ensure that the value f(x) is .The example below shows how you can use this definition to prove a statement about the limit of a specific function at a specified value.Introduction to the Epsilon Delta Definition of a Limit. So then, when I plug these bounds into the last part of your implication, I get 1 / (1+2) = 1/3 < $\epsilon$ I hope this helps.Understanding Epsilon-Delta Proof3 mai 2020Proving limits with epsilon delta for Multivariable Functions10 oct. But, $\epsilon$-$\delta$ is certainly not my speciality).4 Example: a \delta-epsilon proof The kind of problem commonly called a \delta-epsilon proof is of the form: show, using the formal de nition of a limit, that lim x!cf(x) = Lfor some c;f;L. Show that the square root function f(x) = x is continuous on [0,∞).To Acquire a Delta, Fix and Epsilon Example Suppose we are given an >0, and we know that jf(x;y) 0j< for some (x;y).eduRecommandé pour vous en fonction de ce qui est populaire • Avis
Epsilon-Delta Definition of a Limit
I hope this isn't too much, but it's these sorts of problems that I seem to be having the most trouble with. f(4) is de ned and equals.comFurther Examples of Epsilon-Delta Proofocf.Epsilon-delta exercises - Solved Problems on ε-δ Technique . it is clear that.
epsilon-delta definition ultimate introduction

Then we present the forwards implications using the found δ. Suppose x ≥ 0 and > 0.
Limit of a function
My ultimate introduction to the epsilon-delta definition of limits in calculus! The epsilon-delta definition of a limit is commonly considered the hardest to. To prove this I am trying to use the epsilon-delta definition.The intuitive notion of a limit may be converted into a rigorous mathematical definition known as the epsilon-delta definition of the limit. 2015complex analysis - Proof for the existence of a limit .These two statements are equivalent formulations of the definition . Then, whenever 0 < |x − 2| < δ, we have.Critiques : 10 0 < | x − x 0 | < δ | f ( x) − f ( x 0) | < ε.5) Prove that limits are unique. ∀ ε > For any \varepsilon > 0 ε > 0 chosen by Alice, Bob would like to find \delta >0 δ > 0 such that if x x .Exercises - The Epsilon-Delta Definition of a Limit (and Review) Prove limx→−1(5x + 7) = 2 lim x → − 1 ( 5 x + 7) = 2 using the epsilon-delta definition of a limit. The claim to be shown is that for every . However, the steps are usually a bit backward to what the actual definition is. There are four possible limits to define here. limits - $\varepsilon Afficher plus de résultatsBalises :Epsilon-Delta Definition of LimitCalculusFinding Epsilon and Delta Limits Describe the epsilon-delta definitions of one-sided limits and infinite .













