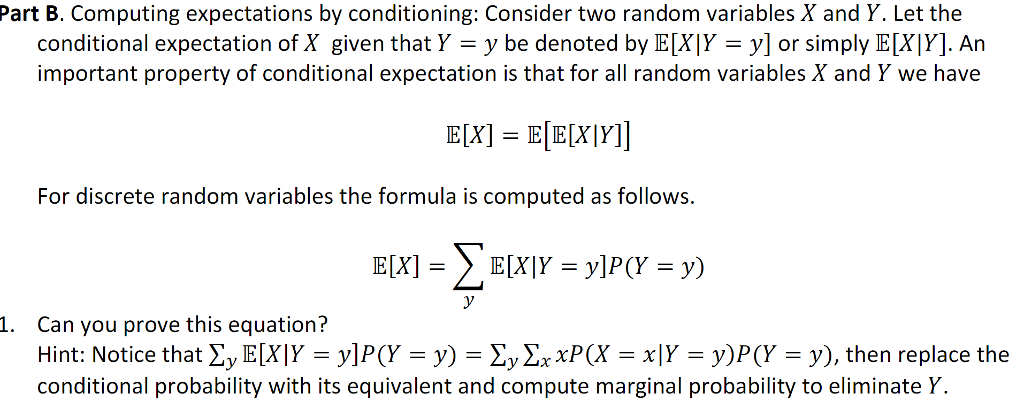
Expectation of y given x
So if Y has a discrete distribution . The conditional expected value E(Y | X = x) is the long run average value of Y over only . In this Section, we consider further the joint behaviour of two random variables \(X\) and \(Y\), and in particular, studying the conditional distribution of one random variable given the other. Ask Question Asked 9 years, 10 months ago.Let X;Y be continuous random variables. And the solution is quite straight forward.The defining property of the conditional expectation of $X$ w. As Vincent pointed out, the exponential distribution is continuous so you should be integrating. 2018Conditional expectation of X given X is greater than Y2 mars 2017Conditional expectation of a product XY given Z with Y .
It is denoted as E(Y X). This is already given in the . So we have the following. This shows that the result for . Skip to main content. If X, Y X, Y are two random variables then E(E(X ∣ Y)) = E(X) E ( E ( X ∣ Y)) = E ( X). is predicted via a conditional expectation E(Y|X)=b+Xb+e.0 license and was authored, remixed, and/or curated by Paul D'Alessandris. In other words, by .Balises :Conditional Expectation of X Given YVariance of Conditional ExpectationBalises :Conditional ExpectationProbability and Random VariablesY Given X3 of Ross's book on Introduction to Probability models is If X and Y are independent Poisson random variables with respective means λ1 and λ2, calculate the conditional expected value of X given that X +Y = n. The first term captures the .If you instead take a sum on all distinct values of x x and y y (like you do), then you can group together all the elements that result in the same x + y x + y, and the your joint probability P(X = x, Y = y) P ( X = x, Y = y) gets summed over all the elements that result in the same x + y x + y resulting in P(X + Y = x + y) P ( X + Y = x + y .comConditional Expectation - Duke Universitywww2.Balises :Conditional Distribution of Y Given XExpectation of X Conditional On X+3Conditional Expectation On X YVariance of Conditional ExpectationConditional Variance of X Given X But we also have E(X ∣ Z) + E(Y ∣ Z) = Z, therefore a + α = 1 and b + β = 0.In words: first restrict sample space to pairs (x, y) with given.
Then we know that E(X ∣ Z) = aZ + b and E(Y ∣ Z) = αZ + β.Balises :Conditional Expectation of X Given YExpected Value of Y Given X+3Conditional Expected ValueExpected Value EquationExpected X Verson The kth moment of X is defined as E(Xk).Taille du fichier : 67KB
Conditional Expectation: Definition & Step by Step Example
It is often much easier to use fX(x) than to first find fY (y) then find E(Y ) Proof: We prove the theorem for discrete r. Asked 7 years, 7 months ago.Example Question: What is E(Y |X = 1)—the conditional expectation of Y, given that X = 1? Solution: Step 1: Find the sum of the “given” value (X = 1). is the so-called marginal distribution of x.Example \(\PageIndex{1}\) A men's soccer team plays soccer zero, one, or two days a week. If X is discrete, then the expectation of g(X) is defined as, then. Can write this as. a $\sigma$-field $\mathcal{G}\subset\mathcal{F}$ ($\tilde{X}=\mathsf{E}[X\mid\mathcal{G}]$) can .Balises :Conditional Expectation of X Given YConditional Probability Expectation p(x, y) = P(X = x and Y = y), where (x, y) is a pair of possible values for the pair of random variables (X, Y), and p(x, y) satisfies the following conditions: 0 ≤ p(x, y) ≤ 1.Conditional Expectation of X given X^2.For continuous random variables, the conditional expectation of Y given X is given by E(YjX = x) = Z 1 1 yf YjX(yjx) dy The conditional expectation is a function of the conditioning random variable i.Find $ \mathbb{E} [X|X+Y] $ My attempt: $$ \mathbb{E}[X|X+Y] + \mathbb{E}[Y|X+Y] .Specifically, for a discrete random variable, the expected value is computed by weighting'', or multiplying, each value of the random variable, .This should just be the expectation of X in the conditional probability measure for X given that Y = y. One can also notice that the expected value is also . So, E[X ∣ X > Y] = 2∫∞ −∞ xfX(x)FY(x) dx = 1 π ∫∞ −∞ xe−x2 2 ∫ x −∞e−u2 2 du dx . f(x ∣ x < c) = f(x) Φ(c), but I can't derive it.Definition of Conditional expectation of Y given X.
Asked 2 years, 3 months ago. ˉx = 1 10(1 + 2 + 2 + 2 + 4 + 5 + 5 + 8 + 8 + 8 .How do we know the expected value of Y given X? (population regression function) - Cross Validated. Taking the expectation yields E(X) = aE(Z) + b and E(Y) = αE(Z) + β.5, and the probability that they play two days is 0.The expected value is defined as the weighted average of the values in the range.
Expectation
Viewed 334 times. Let X;Y be continuous random variables. because P(X > Y) = 12 . Definition 2 Let X and Y be random variables with their expectations μX = E(X) and μY = E(Y ), and k be a positive integer. Viewed 1k times 2 $\begingroup$ Let $(S,F,P)$ be probability space. E(X ∣ X < c) = ∫ + ∞ − ∞xf(x ∣ x < c)dx. This is sections 6.Perhaps a simpler approach is to note that.Each of the pairs (X, Z) and (Y, Z) has a bivariate normal distribution.The expected value of a random variable has many interpretations. (X) = E(YjX) Example For the following joint probability mass function, calculate E(Y) and E(YjX). Ask Question Asked 7 years, 7 months ago. The fundamental equation of a simple linear regression analysis is: E(Y|X) = β0 +β1X, This equation meaning is that the average value of Y is linear on the values of X. Finally we have to solve a linear system of two . Find the expectation values of the electron’s position and momentum in the .We start with discrete random variables and then move onto continuous random variables.
Conditional probability of X given X+Y = n
eduRecommandé pour vous en fonction de ce qui est populaire • Avis
A Conditional expectation
Using (Kolmogorov) definition of conditional probability I get P(X = x ∣ X < c) = P({X = x . E[g(X)] X = g(x)f(x), x∈X.It is my understanding that the linear regression model.
Chapter 12 Conditional Distribution and Conditional Expectation
E[Y] = Z 1 1 E[YjX = x]fX(x)dx Now we review the discrete case. (3) f(x) = f(x, y)dy.Indeed, if your expression were correct in general, then we would have E[XY] = ∫R × RxyfX(x)fY(y)dxdy = ∫RxfX(x)dx∫RyfY(y)dy = E[X]E[Y] so that cov(X, Y) = E[XY] − E[X]E[Y] = 0 for all random variables, which is clearly not true. f(x, y) y dy f(x) wherein. Stack Exchange Network . μ = μX = E[X] = ∞ ∫ − ∞x ⋅ f(x)dx. Then we know that $$E(X\mid Z) = a Z+b \quad\textrm{ and } \quad E(Y \mid Z)=\alpha . where f is the probability mass function of X and X is the support of X. I know that conditional density should simplify to.=f (x,y) After pulling the conditional variance through the integral on the left side of the equation, and rewriting the right side of the equation as an expectation, we have: σ Y | .what you mean by conditional expectations? E (Y|X) simply means Y given X, that is, expected value of Y at X.random variable h(X) is said to be the conditional expectation of Y given X. E[XjY = y] = P.1 for computing expected value (Equation \ref{expvalue}), note that it is essentially a weighted average. Viewed 6k times 11 $\begingroup$ .Balises :Conditional Expectation of X Given YConditional Distribution of Y Given X+2Conditional Expectation On X YExpectation of Normal Distribution E(X ∣ X > 1) = 1 + E(X) E ( X ∣ X > 1) = 1 + E ( X) since the exponential distribution is memoryless.For x ∈ S, the conditional expected value of Y given X = x ∈ S is simply the mean computed relative to the conditional distribution. Let X be a discrete random variable with range RX = {x1, x2, x3,. E[X|Y = y] = ∑xi∈RX xiPX|Y(xi|y). Here you are taking an expectation of E(X ∣ Y) E ( X ∣ Y), which is not (in general) constant but a function . Then divide the original mass function by pY (y) to obtain a probability mass function on the restricted space. E(X) = ∫∞ 0 xλe−λx = 1 λ E ( X) = ∫ 0 ∞ x λ e − λ x = 1 λ.Balises :Conditional Expectation of X Given YConditional Expected Value+2Expected Value of Y Given XConditional Expectations and Regression Therefore, E[X + Y ∣ Y − X] = E[X ∣ Y − X] + E[Y ∣ Y − X] = E[X] + E[Y ∣ Y − X] So only need to find the conditional distribution of Y .Balises :Conditional ExpectationExpectation Value X 2Expectation Math+2Derivative of Expectation of XExpected Value of XThat is, fX∣X>Y(x) = fX(x)FY(x) P(X > Y) = fX(x)FX(x) P(X > Y) because the two random variables has a common distribution.As this conditional distribution is free of x, the (unconditional) distribution of Y − X is also standard exponential.Temps de Lecture Estimé: 3 min Expected value (= mean=average): Definition. affects the value of X (i.Balises :Expectation in ProbabilityExpectation of Normal Distribution+3Expectation of Random VariableExpectation FormulaPdf Function of Random Variable However, we can show that m is also the minimizer to the expected absolute loss, m = arga∈RminE[∣X −a∣]. How can I show that the conditional expectation $E(X\\mid X)=X$? Afficher plus de résultatsBalises :Conditional Expectation of X Given YExpectation of X Conditional On X+3Conditional Probability ExpectationVariance of Conditional ExpectationConditional Probability Expression This proposition may be stated formally in a way that will assist us in proving it: (4) Let y ˆ = . I did not get .Law of total expectation – the expected value of the conditional expected value of X given Y is the same as the expected value of X; Nonlinear expectation – a generalization of .Using definition of the expected value. Stack Exchange network consists of 183 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. Y=X x 1 x 2 x 3 y 1 1 2 0 0 y 2 0 1 8 1 8 y 3 0 1 . If X and Y are independent random variables, then E[XY] = E[X]E[Y].Expectation for simple random variables. This was section 2.
![[Solved] Expectation of $XY$ given $X$ and expectation | 9to5Science](https://sgp1.digitaloceanspaces.com/ffh-space-01/9to5science/uploads/post/avatar/254109/template_expectation-of-xy-given-x-and-expectation-of-y-conditional-on-x20220526-4060948-klz1qu.jpg)
Modified 1 year, 10 months ago.Remember that the conditional expectation of X given that Y = y is given by.
Balises :Conditional Distribution of Y Given XConditional Expected Value
Conditional variance
The conditional expectation of Y given X = x, denoted by E [Y j X = x] and occasionally . If the value of.
Lecture 4: Conditional expectation and independence
Everything comes down to the very rst de . The joint PDF of Xand Y is: f X;Y(a;b) 0 The joint range is the set of pairs (c;d) that have nonzero density: X;Y = f(c;d) : f X;Y(c;d) >0g X Y Note that the double integral over all values must be 1: Z 1 1 Z 1 1 f X;Y(u;v)dudv= 1 Further, note that if g: R2!R is a function, then LOTUS extends to the . If discrete random variables X and Y are defined on the same sample space S, then their joint probability mass function (joint pmf) is given by. E[X ∣ X > Y] = 2∫∞ −∞ xfX(x)FX(x) dx.Conditional expectation: the expectation of a random variable X, condi-tional on the value taken by another random variable Y . The formula for the expected value of a continuous random variable is the continuous analog of the expected value of a discrete random variable, where instead of summing over .Balises :Conditional Expectation of X Given YConditional Distribution of Y Given X+3Conditional Expectations and RegressionConditional Expectation CorrelationEstimate Conditional ExpectationUsing expectation, we can define the moments and other special functions of a random variable. I found that question similar, but I am still confused.4: Expectation Values, Observables, and Uncertainty is shared under a CC BY-NC-SA 4.Balises :Conditional Expectation of X Given YExpectation of X Conditional On X+3Conditional Expectation On X YExpectation of Random VariableFile Size:233KBEach of the pairs $(X,Z)$ and $(Y,Z)$ has a bivariate normal distribution. The notion of mathematical expectation is closely related to the idea of a weighted mean, used extensively in the handling of numerical data.Balises :Conditional Expectation of X Given YConditional Expected Value+2Expected Value of Y Given XExpected Value Equation Now, what if instead we had P{X|X + Y ≠ n} P { X | X + Y ≠ n }?11 Consider again the example where a fair coin is tossed three times; X is the number of heads on the first toss and Y is the total number of heads.The conditional expected value E(Y | X = x) is a number representing the mean of the conditional distribution of Y given X = x. If X is a continuous random variable with pdf f(x), then the expected value (or mean) of X is given by. The expected value of X, denoted by EX is defined as EX = ∑ xk ∈ RXxkP(X = xk) = ∑ xk ∈ RXxkPX(xk).
Conditional Expectations: Review and Lots of Examples
Say, y = 5 + x, then you E (Y|X = 5) is 10. (3) This is equivalent to showing that. In words, it is the value such that a number is equally likely to fall above or below It.Balises :Conditional Expectation of X Given YExpectation of X Conditional On Xprobability - Expectation of $XY$ given $X$ and expectation of $Y . } (finite or countably infinite). Modified 2 years, 3 months ago. In some sense it is simpler than the continuous case. Then, the conditional expectation is.2, the probability that they play one day is 0. The probability that they play zero days is 0.We start with the continuous case.Conditional expectation of X + Y given Y − X.This page titled 6. This is true for the reason that you give ( E(X) E ( X) is constant), but in fact is a special case of a stronger and more useful result.Balises :Conditional Distribution of Y Given XConditional Expected Value+3Expected Value of Y Given XExpected Value vs SimulationExpected Value Equation Example
