Fisher information cramer rao
Fisher information and Cramér–Rao planes have been identified as useful and versatile tools for characterizing complex systems, see e.
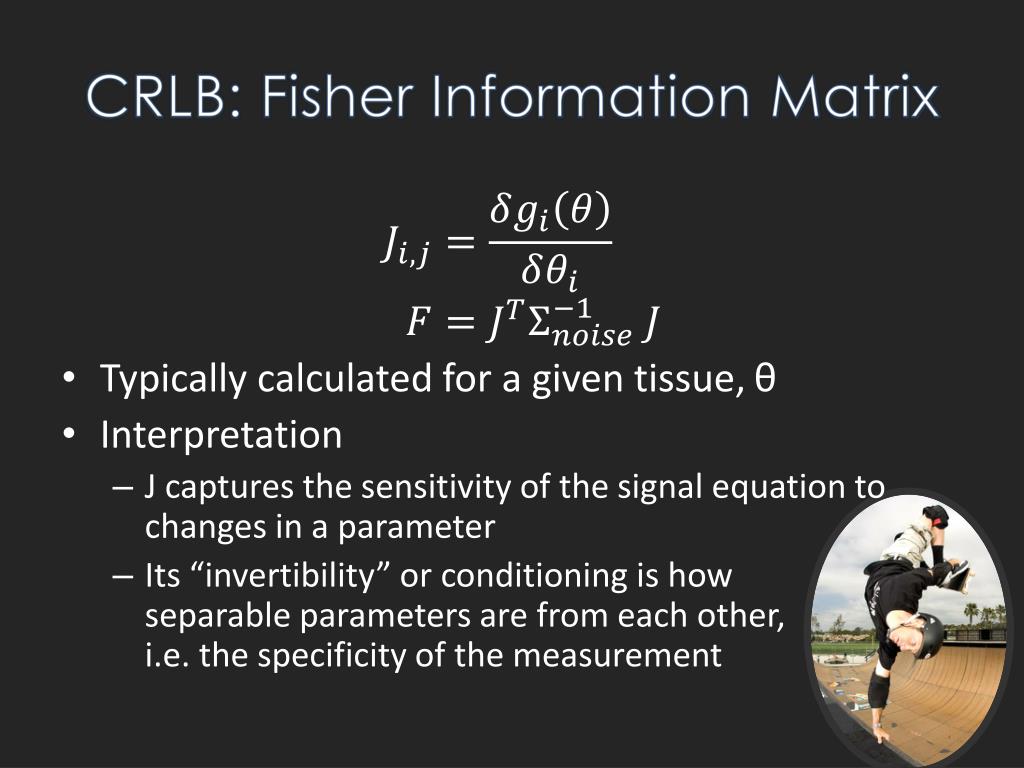
The Fisher information and the quantum Cramer-Rao sensitivity limit of continuous measurements. However, it is . IEEE Transactions on Automatic Control, 40 (8) (1995), pp.The fisher has a weasel-like body, bushy tail, tapered muzzle, and low rounded ears.The Cramér-Rao lower bound (CRLB) is widely used in the design of magnetic resonance (MR) experiments for parameter estimation. Søren Gammelmark, Klaus Mølmer. An open issue is the possible convexity property of the generalized . Previous work has considered only Gaussian or Rician noise distributions in this calculation.
Information de Fisher — Wikipédia
Asked 12 years, 11 months ago.
Fisher信息量与Cramer-Rao不等式
Adults are usually 50–63 cm (20–25 inches) long, excluding the 33–42-cm . A Cramer–Rao bound for multi-dimensional discrete-time dynamical systems. Fisher Electronics, a Sanyo subsidiary producing hi-fi equipment.在参数估计和统计中，Cramer-Rao界限（Cramer-Rao bound, CRB）或者Cramer-Rao下界（CRLB），表示一个确定性参数的估计的方差下界。命名是为了纪念Harald Cramer和Calyampudi Radhakrishna Rao。这个界限也称为Cramer-Rao不等式或者信息不等式。它的最简单形式是：任何无偏估计的方差至少大于Fisher信息的倒数。
statistics
Sir Ronald Aylmer Fisher (born February 17, 1890, London, England—died July 29, 1962, Adelaide, Australia) was a British statistician and geneticist who pioneered . We consider the class of random compression matrices that satisfy a version of the Johnson-Lindenstrauss lemma, and we derive .1 of Hardle and Simar.Irving Fisher (born February 27, 1867, Saugerties, New York, U. 如果一个无偏估计的方差达到了Cramér-Rao bound ，那么就说这个估计器是efficient的。.ARTICLE OPEN Quantum Fisher information measurement and verification of the quantum Cramér–Rao bound in a solid-state qubit Min Yu 1, Yu Liu , Pengcheng Yang , Musang Gong , Qingyun Cao1,2 . View in Scopus Google Scholar. We consider the class of random compression matrices whose distribution is right-unitarily . 这个Cramér-Rao bound 主要说明的是无偏估计器的方差下界，也就是去估计一个真实值，得到的测量值的方差的最小值，Cramér-Rao bound 就说明了这个方差最小为多少。. So, I made a mistake, but I can't see it. Hot Network Questions What's the most common word in Latin? NSF grant proposal not reviewed despite being received Academic view of graduates who go to US national labs? . On considère donc une famille de variables aléatoires (Xθ)θ∈I ( X θ) θ ∈ I dépendant d'un paramètre θ θ qui peut parcourir un intervalle I I (penser par exemple à une loi de Poisson, etc. inequality fails, then the conditions of theorem does not fulfilled.Taille du fichier : 119KB
Intuitive explanation of Fisher Information and Cramer-Rao bound
So in this case,the CR lower bound is not reached, but as I said above, with the other definition of the Gamma distribution it worked. 2014 May 2;112(17):170401.
Lecture 23: Cram er-Rao and Uninformative Priors 23-3 23.3 Remarks We note the following points with respect to Cramer-Rao Lower Bound (CRLB).Overview
Borne de Cramér-Rao — Wikipédia
In this paper, we analyze the impact of compression with complex random matrices on Fisher information and the Cramér-Rao Bound (CRB) for estimating unknown parameters in the mean value function of a complex multivariate normal distribution.本文我们主要想讨论Fisher信息量及Cramer-Rao不等式，这里就与上面提到的有效性有关系。 提到有效性，我们自然有这样一个想法，就是希望估计量的方差愈小愈好.1103/PhysRevLett. Fisher information is a central concept in the theory of maximum likelihood (ML) inference, and much of the chapter is aimed at ., a football club in England. Instructor: Songfeng Zheng. Let (y; θ) be the joint density of random vector of observations Y ×1 with unknown parameter vector θ ×1 The likelihood is defined as (θ) = (Y; θ) .
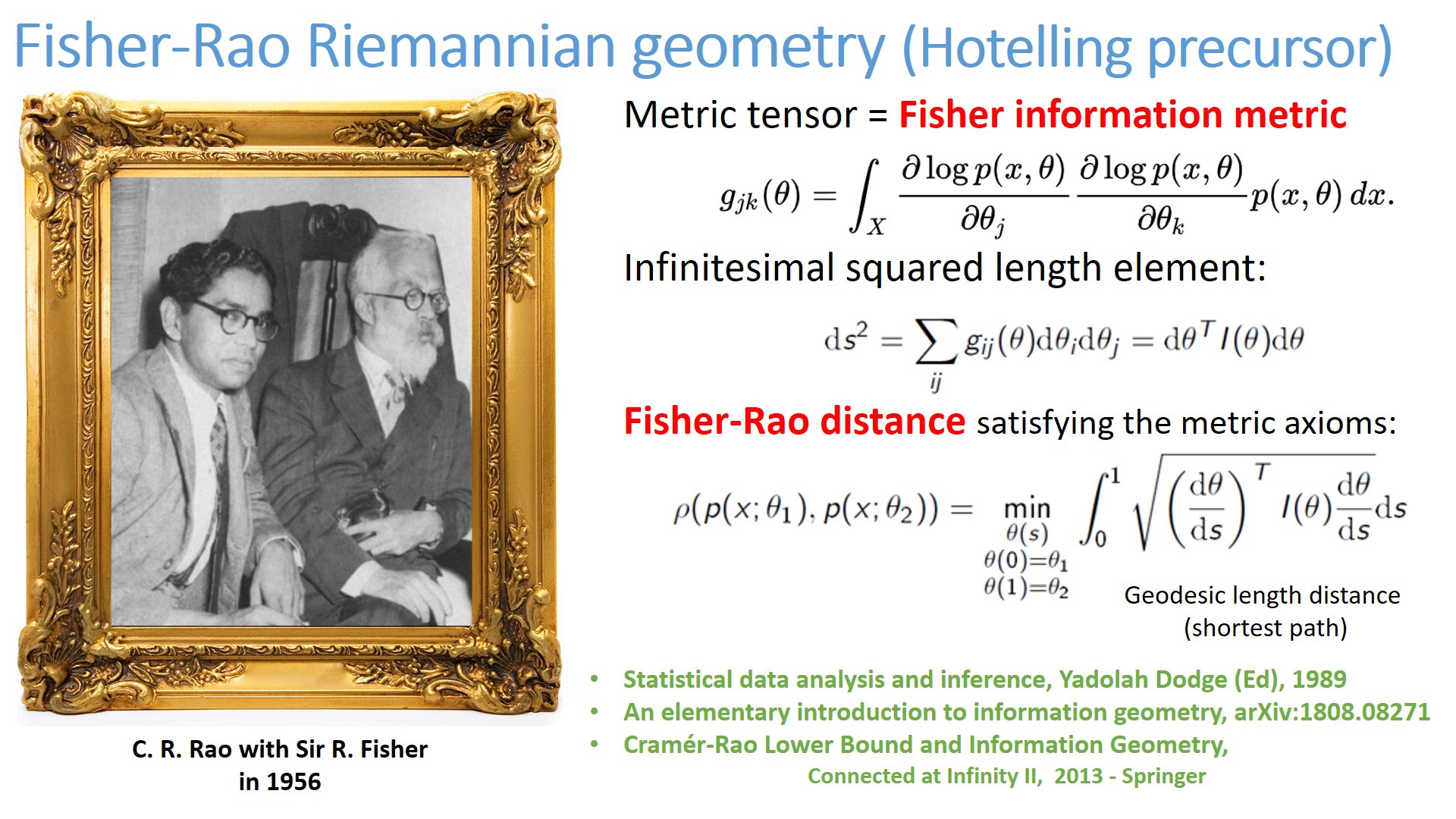
Maybank Department of Computer Science and Information Systems, Birkbeck College University of London, London, UK Synonyms Rao metric Related Concepts Cramér-Rao Lower Bound Fisher Information Hough Transform Kullback-Leibler Divergence Maximum Likelihood Estimation Riemannian Metric .Réponse 1 : avec les conditions de régularités du début + une autre, l’estimateur du maximum de vraisemblance vérifie.
Bornes de Cramér-Rao intrinsèques et matrices de covariance
Vue d’ensemble if condition
Fisher-Rao Metric
This can be extremely useful in several ways: If we nd an estimator that achieves the CRLB, then we know that we have found a \minimum variance unbiased estimator (MVUE)! The CRLB can provide a benchmark against .L'inégalité de Cramer-Rao est une inégalité portant sur la variance des estimateurs en statistique. In other cases (e. For instance, the Cramér–Rao inequality has been applied to the study of uncertainty principles in quantum mechanics, and to the analysis .
Cramér
Is we have at least one estimate s.
Cramér-Rao bound(一)
First published: 22 July 2011. Book Author (s): Russell B. In contrast to random errors with a .Jianning Li, Haodi Liu, Zhihai Wang, Xuexi Yi.It is the Cramér-Rao (CR) inequality that establishes the minimum variance that can be achieved by unbiased estimators, and the key concept in the statement of this inequality is the expected Fisher information.In this paper, we analyze the impact of compressed sensing with random matrices on Fisher information and the CRB for estimating unknown parameters in the mean value function of a multivariate normal distribution.this gives: N2α β2 = (Nα β2)−1 N 2 α β 2 = ( N α β 2) − 1.
On generalized Cramér
For this reason, the concept is applicable for random measurement errors. Cramer–Rao bounds for discrete-time nonlinear filtering problems.The Cramér–Rao inequality gives a lower bound for the variance of an unbiased estimator of a parameter.The quantum Cramér–Rao bound sets a fundamental limit on the accuracy of unbiased parameter estimation in quantum systems, relating the uncertainty in determining a . Elle est définie comme l'espérance de l'information .
The Fisher (electronics manufacturer), a line of electronics marketed by . which is not true, because: N2α β2 > β2 Nα N 2 α β 2 > β 2 N α. Equality holds in the previous theorem, and hence h(X) is an UMVUE, if and only if there exists a function u(θ) such that (with probability 1) h(X) = λ(θ) + u(θ)L1(X, θ) Proof. Note that now we switch our attention from distribution of Y to function of θ where Y (data) is held fixed/known. Borne de Cramér-Rao bayésienne et hybride. In the parameter estimation problems, we obtain information about the parameter from a . Available for US customers .En statistique, l' information de Fisher quantifie l'information relative à un paramètre contenu dans une distribution.Cramér-Rao Inequality and Fisher Information. Attention : Un estimateur efficace n'EXISTE pas toujours!!! (à cause des conditions de Cramer-Rao) Sans biais de variance minimale => efficace. Epub 2014 Apr 28.Fisher-Rao Metric Stephen J. Both conditions on p(x; ) are necessary for the bound to hold. After that it is meaningless to check this inequality further for any other estimate: regularity is absent, Fisher information is .Each derived corresponding Fisher information shows a characteristic behavior with respect to the measurement time. 那么能够小到什么程度呢？也就是有没有下界？什么条件下方差下界存在？下面就讨论建立一个方差 . Reading: Section 6.Fisher information and the Cramér-Rao lower bound in single-pixel localization microscopy with spatiotemporally modulated illumination J Opt Soc .The Fisher information is used in machine learning techniques such as elastic weight consolidation, which reduces catastrophic forgetting in artificial neural networks. For example, condition 1 does not hold for the uniform distribution U(0; ) and hence the CRLB is not valid. An estimator of λ that achieves the Cramér-Rao lower bound must be a uniformly minimum variance unbiased estimator (UMVUE) of λ. Réponse 2 (formule de Slepian-Bang) : si les observations sont gaussiennes à moyenne et covariance paramétrées. De plus, sa relation avec la borne Cramer-Rao ne m'est pas apparente.
Fisher Information and Cram¶er-Rao Bound
The quantum Cramér–Rao bound sets a fundamental limit on the accuracy of unbiased parameter estimation in quantum .Intuitive explanation of Fisher Information and Cramer-Rao bound.1002/9780470094846. Precision measurements with quantum systems rely on our ability to trace the differences between experimental signals to variations in unknown physical parameters. Call us at (888) 823-9566. [29, 38, 39], and it would therefore be interesting to look at the potential benefits of using the extended versions in such problems.
Fisher information
The Fisher information matrix .The Fisher Information Matrix (FIM) and the Cramér-Rao Bound (CRB) are powerful tools for quantifying uncertainty in multi-parameter models., regarding the estimation of multiple unknown quantities with .—died April 29, 1947, New Haven, Connecticut) was an American economist best known for his . Quelques remarques sur les bornes de Cramér-Rao.
vérifiant l'ensemble des conditions de Cramer-Rao.The concept of Fisher information is based on a (known) probability density function referred to as the likelihood function of the signal to be evaluated. Fisher information and the quantum Cramér-Rao sensitivity limit of continuous measurements Phys Rev Lett.Fisher information, in conjunction with the Cramér–Rao inequality, and Rao's distance, are now important tools in the study of the foundations of both quantum mechanics and statistical physics.The Cramer-Rao Lower Bound.Cramer - Rao inequality stated that for regular distributions, the inequality holds for any unbiased estimate.
Inégalité de Cramer-Rao
Fisher Information and Cram¶er-Rao Bound. Get the Fisher App.Confusion about Fisher information and Cramer-Rao lower bound.Download PDF Abstract: Precision measurements with quantum systems rely on our ability to trace the differences between experimental signals to variations in unknown physical parameters.Legal Information Legal Information Connect With Us.
