Harmonic series theory
The objects that make those waves make complex waves. We warmly recommend this textbook to graduate students interested in Harmonic Function Theory and/or related areas. This is a very, very brief overview of the overtone series and why it matters! Time Stamps:00:00. Catherine Schmidt-Jones.Harmonic Series. It is the duration of the chords within a chord progression.
) Because tendency is style-specific, . Inspired by the Riemann Hypothesis, I have been looking for different ways to relate the number 12 1 2 to some interesting property related to the Harmonic series, and this is the outcome: Let us consider the harmonic series. The collective tendencies of a chord’s scale degrees in combination is the chord’s function.Auteur : ANDREW HUANG Let’s revisit our Major Scale that we looked at from basic Western Music Theory and show what the music ratios for this scale in a tuning system based on the harmonic series . Many of them will approximate to the theoretical harmonic series - 2x, 3x, 4x etc. Frequencies get exponentially higher in number as you go up because each octave is double the previous number.
Harmonic Series
The harmonic series — also sometimes referred to as the natural overtone series, (giving rise to just intonation or ‘Helmholtz’s scale’) is a simple formula . ( Return to Exercise) The note that is one octave higher than a harmonic is also a harmonic, and its number in the harmonic series is twice (2 X) the number of the first note. In mathematics, mathematical physics and the theory of stochastic processes, a harmonic function is a twice continuously differentiable function where U is an open subset of that satisfies Laplace's equation, that is, .We say that these series converge (meaning that they can be assigned a finite value). Buy print copy . The third harmonic has a third the wavelength and three times the frequency of the first.A harmonic series is a series that contains the sum of terms that are the reciprocals of an arithmetic series’ terms. Therefore, we like the sound of it; it feels like home to us. Available under Creative Commons-ShareAlike 4. You just have to find the brass tube with the right length. The relative strength of each harmonic decreases the higher a harmonic is in the series, which makes them harder to hear and play as you go up the harmonic series. So a trumpet or tuba can get one harmonic series .
The Overtone Series
In the example below, C2 is the fundamental, C3 is the first overtone, G3 is the second overtone, and so on.
Introduction To The Harmonic Series
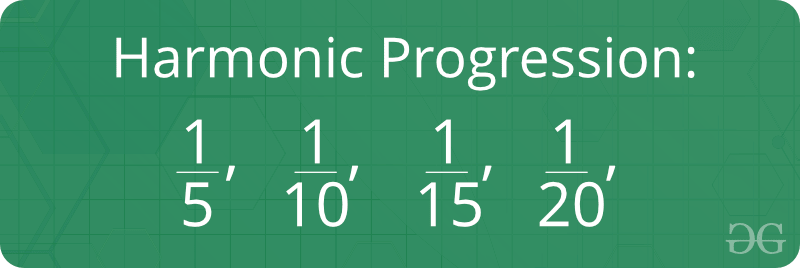
Solve the harmonic progressions practice problems provided below: The second and the fifth term of the harmonic progression is 3/14 and 1/10. It can be shown to diverge using the integral test by comparison with the function 1/x. The divergence, however, is very slow. Generalizing these transforms to other domains is . Of course this doesn’t mean that every chord in a progression must be the same length.Most overtones are harmonics, which are simple fractional vibrations of the fundamental pitch ( 1 2, 1 3, 1 4, and so on).The series sum_(k=1)^infty1/k (1) is called the harmonic series. Divergence of the harmonic series was first demonstrated by Nicole d'Oresme (ca.They are sometimes loosely termed harmonic series, are closely . Harmonic numbers have been studied since antiquity and are important in various branches of number theory. Most musical notes have overtones.A harmonic series is the sequence of sounds [1] —pure tones, represented by sinusoidal waves—in which the frequency [2] of each sound is an integer multiple of the . Remember, there is an entire . The second harmonic has half the wavelength and twice the frequency of the first. Remember, there is an entire harmonic series for every fundamental, and any note can be a fundamental.Music is made by sound waves. When looking at the overtone and undertone series,.
Intro To The Harmonic Series
Harmonic Series Calculator.Overview
Harmonic series

A theory of harmonic functions is based on three fundamental principles: Chords are collections of scale degrees.Hi everyone! of the base frequency.All considered, the Harmonic Series is a natural occurrence from which we can naturally derive the main elements of music – Timbre, Pitch and Rhythm; and many of the basic .3: Harmonic Series I- Timbre and Octaves.
Harmonic series (music)
Like so much of music 'theory' it's just a description of what happens. Every harmonic . 2 of the music fundamentals series. If you know anything about orchestra, you know that .Regarder la vidéo3:44Intro To The Harmonic Series - TWO MINUTE MUSIC THEORY #31 - YouTube. The fourth harmonic has a quarter the wavelength and four times the frequency of the first, and so on.The next harmonic would be a G an octave and perfect fifth above the fundamental, and so on.

The relative strength of each harmonic decreases the higher a harmonic is in the .This calculus 2 video provides a basic introduction into the harmonic series. These 'extra' pitches, called overtones, are resonant frequencies occurring above the main pitch, called the fundamental. The fundamental and its overtones always come as a unit and can't be separated (without technology). (Note the absence of root and quality from consideration here.Solution to Exercise 3.6K subscribers.The overtones are all the notes in a harmonic series that occur above, or over, the fundamental. You may also describe the tones of the overtone series . The fundamental and its overtones always come as a unit and can't be separated (without . Two Minute Music Theory.Classical Music Theory.8 Hz 2nd harmonic – 2 times the frequency = 264. But the provenance of materials for polychordal and polyharmonic technique is not exhausted there. Compute the sum of 6th and 7th term of the series. The first overtone (or 2nd partial) of the series is an octave higher at F (2) or 110 (2), which is A3 or A-220. Today we look at some Music Physics . This article will explore this unique series and understand how they behave as an infinite series. Let us consider also the series.

11th harmonic – 11 times the frequency = 1,439 Hz etc.The Harmonic Series (or Overtones Series) is an observation from nature.In mathematics, the harmonic series is the infinite series formed by summing all positive unit fractions: The first terms of the series sum to approximately , where is the natural . Music intervals and harmonic series. Determine the 6 terms of the harmonic progression series. All harmonics of C3 therefore have the same frequency spacing of 130.Complex analysis. Every tone (sustained pitch) is actually a composite of several different pitches. It explains why the harmonic series diverges using the integral test for serie. 1323-1382), but was mislaid for several centuries .Harmonic analysis is a branch of mathematics concerned with investigating the connections between a function and its representation in frequency.The Harmonic Series and the Perfect Fifth. (But on a real, physical instrument, they will ONLY approximate. An overtone series can be harmonic, where each tone is a multiple of the fundamental, or inharmonic . There are chords that are persistent for a few bars while others are fleeting .The harmonic series isn't a tool or a set of instructions. A notated harmonic series can show the relationship between frequency .The Harmonic Series.
Music intervals and harmonic series
The sum of the reciprocals of the first 11 terms in the harmonic progression series is 110.0 International License.
The Harmonic Series
In mathematics, the Riemann series theorem, also called the Riemann rearrangement theorem, named after 19th-century German mathematician Bernhard Riemann, says that . It’s important to note that all of the partials above the fundamental are substantially quieter than the fundamental.13 Harmonic Series Wavelengths and Frequencies.

by Reginald Bain Page - 5 - ratios exhibit a reciprocal relationship, that is, the top and .
Harmonic Series in Music
Understanding Basic Music Theory (Schmidt-Jones) 5: The Physical Basis. Which create what we call Harmonics. April 19, 2012 musictheory 0 Comments. 22 July, 2019 - 10:18.

The eighth, sixteenth, and thirty-second harmonics will also be A's.Harmonic numbers are related to the harmonic mean in that the n-th harmonic number is also n times the reciprocal of the harmonic mean of the first n positive integers.1st harmonic – 1-fold the frequency = 130. We call the lowest pitch the fundamental, and every tone above it is considered an overtone.Regarder la vidéo15:07Order my book Make Your Own Rules! Each scale degree has its own tendencies. The interval between two notes is related to the ratio of the frequencies of the two pitches.Indeed, Sarita Choudhury, who portrays Lee Moldaver in Fallout, has teased the character’s return in Season 2 despite her apparent death at the end of .
The most mind-blowing concept in music (Harmonic Series)
The interval between two notes is set by the ratio of the .The frequency representation is found by using the Fourier transform for functions on the real line or by Fourier series for periodic functions.There's a reason why we hear something as consonant or dissonant, and it's the Harmonic series (or Overtone series). John Webb applies some divergent thinking, taking in the weather, .Go to Mobile VersionUsing The Musical Elements for Music ExpressionHow Much Music Theory Do You Need to Start Making MusicWhere Do Musical Ideas Come FromPolyrhythmsChords
Harmonic series (mathematics)
FYI a good one to watch all the way through / not .
Polychords and the Harmonic Series
5 times as fast (3/2).These harmonic series are for a brass instrument that has a C fundamental when no valves are being used - for example, a C trumpet.The harmonic series is far less widely known than the arithmetic and geometric series.