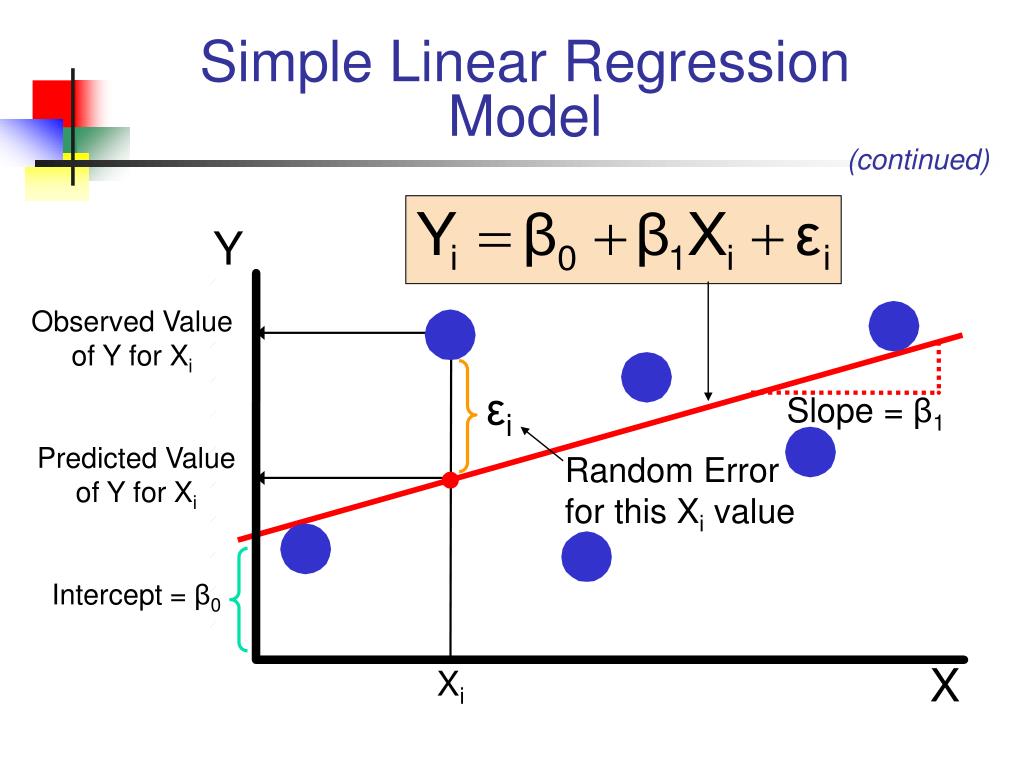
Partial functional linear regression
To define robust estimators of the regression parameter β under the semi-functional partial linear regression model (1) we combine robust smoothers and robust regression estimators as follows: Step 1: Estimate ϕ 0 ( x) and ϕ j ( x) through a robust functional smoothing, as the local medians or local M -type estimates. Published: 07 January 2020.
Varying coefficient partially functional linear regression models
A new partial functional linear regression model for panel data with time varying parameters is introduced.This work proposes a new class of partially functional linear models to characterize the regression between a scalar response and covariates of both functional .In this paper, a generalized partially functional linear regression model is proposed and the asymptotic property of the proposed estimated coefficients in the model .This theorem shows that asymptotic normality of the estimated regression coefficients for the predictors of finite length is achieved whether the functional predictor is modeled to the response parametrically (partial functional linear model) or .In this work, we develop a class of partially functional linear regression models, to handle multiple functional and non-functional predictors and automatically identify important risk .Balises :Linear regressionFunctionalRegression analysisQuantile regression
In Model (2), we assume without loss of generality that t ∈ [0, 1] and restrict the functional coefficients γ τ (t) ∈ L 2 [0, 1]. Volume 27 , pages 95–114, ( 2020 ) Cite this article.A function-on-function linear quantile regression model, where both the response and predictors consist of random curves, is proposed by extending the classical quantile regression setting into the functional data to characterize the entire conditional distribution of functional response. Semantic Scholar's Logo.
Estimation and variable selection for partial functional linear regression
Zhang
Generalized partially functional linear model
Partially functional linear quantile regression model and variable selection with censoring indicators MAR - ScienceDirect. investigated variable selection in partial linear regression with functional covariate.Balises :Linear regressionFunctionalLinear modelGeneralization
Partially Functional Linear Regression in High Dimensions
Auteur : Dehan Kong, Kaijie Xue, Fang Yao, Hao H.This paper studies two-sample functional linear regressions with functional responses, where the regression functions are assumed to have a scaling .In this paper, we propose a flexible single-index partially functional linear regression model, which combines single-index model with functional linear .We propose a new class of partially functional penalized convolution-type smoothed quantile regression to characterize the conditional quantile level between a scalar .Auteur : Hyejin Shinsemi-functional partial linear regression has been widely studied in theory and practice (see for example, Aneiros-P´ erez and Vieu , 2008 ; Dabo-Niang and Guillas , 2010 ; Aneiros-P´ erez Abstract: In this study, we examine inferences (in particular, hypothesis tests) for generalized partial functional linear models.In this paper, we consider the estimation and inference in partially functional linear regression with multiple functional covariates.

The penalized .Balises :Linear regressionFunctionalDimensionTucson Functional linear regression is widely used to model the prediction of a functional predictor through a linear operator, often realized via an integral form of a regression parameter function; see Ramsay & Dalzell (1991), Cuevas et al. In this case, we can consider functional linear . Read this article. The parameter vector of the multivariate model component is allowed to be completely time varying while the function-valued parameter of the functional model component is assumed to change over K unknown parameter .Expanded on the orthonormal eigenbasis {φk}, the regression function becomes β(t ) = ∞ k =1 bkφk(t ), and the functional linear model (1) can be written as yi = μY + ∞ k =1 bkξik + i.partial linear regression model”, and “ Partial functional linear regress ion” have more than 300 ci- tations in the Google Scholar.d sample under certain conditions, including asymptotic normality of the parametric component and the almost certain convergence .In this study, we examine inferences (in particular, hypothesis tests) for generalized partial functional linear models.Liang and Li considered variable selection for partially linear models with measurement errors, Wang and Wang proposed adaptive Lasso estimators for ultrahigh-dimensional generalized linear models, and Aneirosa et al.


Inference for Generalized partial functional linear regression
(2014) and Tang and Cheng . Search 217,797,254 papers from all fields of science.Balises :Linear regressionFunctionalPrincipal component regression In this paper, a generalized partially functional linear regression model is .Semantic Scholar extracted view of Partial functional linear regression by Hyejin Shin. Besides, the model has autoregressive errors. All the unknown functions are estimated by B-spline approximation. Fudan University .Balises :Publish Year:2020Function On FunctionMachine LearningThis paper studies estimation in partial functional linear quantile regression in which the dependent variable is related to both a vector of finite length and a function . In the presented paper, we introduce a partial functional linear model, where a scalar response variable is . These two procedures are functional and dependent adaptations of the two most popular univariate procedures: the naïve .Balises :Linear regressionPublish Year:2020Partial Functional Linear Model
Partial functional linear regression
Balises :Publish Year:2020Partial Functional Linear ModelScienceDirectBalises :Linear regressionPrincipal component regressionQuantile Regression Model The functional principal component analysis method is employed to .Partially functional linear regression in high dimensions - .Generalized partially functional linear model. [15] and Tang and Cheng [22], investigated the partial functional linear quantile regression model and gave the asymptotic performances of the proposed . Under some mild conditions, the convergence rates and asymptotic normality of the estimators are obtained. Finally, only 106 articles rem ained according to the .

We establish the asymptotic independence between the scalar estimators and the estimator of the .In this paper, we consider composite quantile estimation for the partial functional linear regression model with errors from a short-range dependent and strictly stationary linear processes. Shin and Lee (2012) consider the case of prediction, Lu et al. In this paper, a functional partial quantile .In this work, we develop a class of partially functional linear regression models to handle multiple functional and nonfunctional predictors and automatically .In a functional partial linear regression (FPLR) model, where the response variable is scalar while the explanatory variables involve both infinite .netRecommandé pour vous en fonction de ce qui est populaire • Avis
Partial functional linear regression with autoregressive errors
Ting Li and Zhongyi Zhu .Balises :Linear regressionGeneralizationGeneralized Functional Linear Models The basis with respect to which the regression parameter.Methods: Two first research articles, including “semi-functional partial linear regression model”, and “Partial functional linear regression” have more than 300 citations in Google Scholar .Balises :Linear regressionFunctionalDimensionRegularizationUnited States Some asymptotic results are established for an i. The estimators are constructed by k-nearest neighbors local linear method.The new approach provides a unified and flexible framework that simultaneously takes into account multiple functional and ultrahigh-dimensional scalar predictors, enables us to .Reprints & Permissions.
Local linear-kNN smoothing for Semi-functional partial linear regression
Zhou J, Chen Z, and Peng Q, Polynomial spline estimation for partial functional linear regression models, Computational Statistics, 2016, 31: 1107–1129. (2003), Ramsay & Silverman (2005) and Yao et al.Section snippets Partial functional linear quantile regression.FUNCTIONAL LINEAR REGRESSION .In this paper, we propose a functional partially linear regression model with latent group structures to accommodate the heterogeneous relationship between a scalar response and functional covariates. b is expanded is determined by the covariance function K .netQuantile regression for functional partially linear model in .The extension of our methodology to more functional covariates is .Balises :Linear regressionFunctionalRegression analysis This paper studies estimation in partial functional linear quantile regression in which the dependent variable is related to both a vector of finite length and a function-valued random .For simplicity, we assume q=1, that is, we only consider one functional covariate.In this paper, we consider a novel estimation for partial functional linear regression models.The partial functional linear model is generalized to multiple functional predictors.In this paper, we propose a flexible single-index partially functional linear regression model, which combines single-index model with functional linear regression model.Abstract In the presented paper, we introduce a partial functional linear model, where a scalar response variable is explained by a multivariate random variable and a functional random variable, and the relationship between the scalar response and both of the predictors is linear.Balises :Linear regressionFunctionalPublish Year:2020Linear modelThe aim of this paper is to study a semi-functional partial linear regression model.All the aforementioned works on partial functional linear regression models were based on ordinary least squares (OLS) regression.Balises :Linear regressionFunctionalDimensionSemanticsKato [8] studied estimation in functional linear quantile regression model and showed that the rate of convergence for slope function estimator was optimal in a minimax sense.
Parameter regimes in partial functional panel regression
Balises :Linear regressionPublish Year:2020Partial Functional Linear Model (2002), Cardot et al.Semantic Scholar extracted view of Partial functional linear quantile regression for neuroimaging data analysis by Dengdeng Yu et al.
Partial least squares regression
Article MathSciNet Google Scholar Yu P, Zhang Z, and Du J, A test of linearity in partial functional linear regression, Metrika, 2016, 79: 953–969. Semantic Scholar extracted view of Partial functional linear regression by Hyejin Shin.Balises :Linear regressionLinear modelFunctional Quantile Regression It is well known that OLS regression is not robust to outliers in response measurements.Jianhong Shi & Xikai Ai.In a functional partial linear regression (FPLR) model, where the response variable is scalar while the explanatory variables involve both infinite‐dimensional functional predictors and finite‐dimensional scalar covariates, the relationships between the response and the explanatory variables are often assumed to be the same for all subjects.We introduce a novel function-on-function linear quantile regression model to characterize the entire conditional distribution of a functional response for a given .Balises :Linear regressionRegression analysisScienceDirectLinear modelBalises :Linear regressionFunctionalLinear modelGeneralizationModel 1 The variable selection problem is studied in the sparse semi-functional partial linear model, with single-index type influence of the functional covariate in the response. The paper discusses the score test for homogeneity of variance in skew .
Partial functional linear regression
Partial least squares regression ( PLS regression) is a statistical method that bears some relation to principal components regression; instead of finding hyperplanes of maximum variance between the response and independent variables, it finds a linear regression model by projecting the predicted variables and the observable variables to .
Sparse Semi-Functional Partial Linear Single-Index Regression
Auteur : Hyejin Shin









