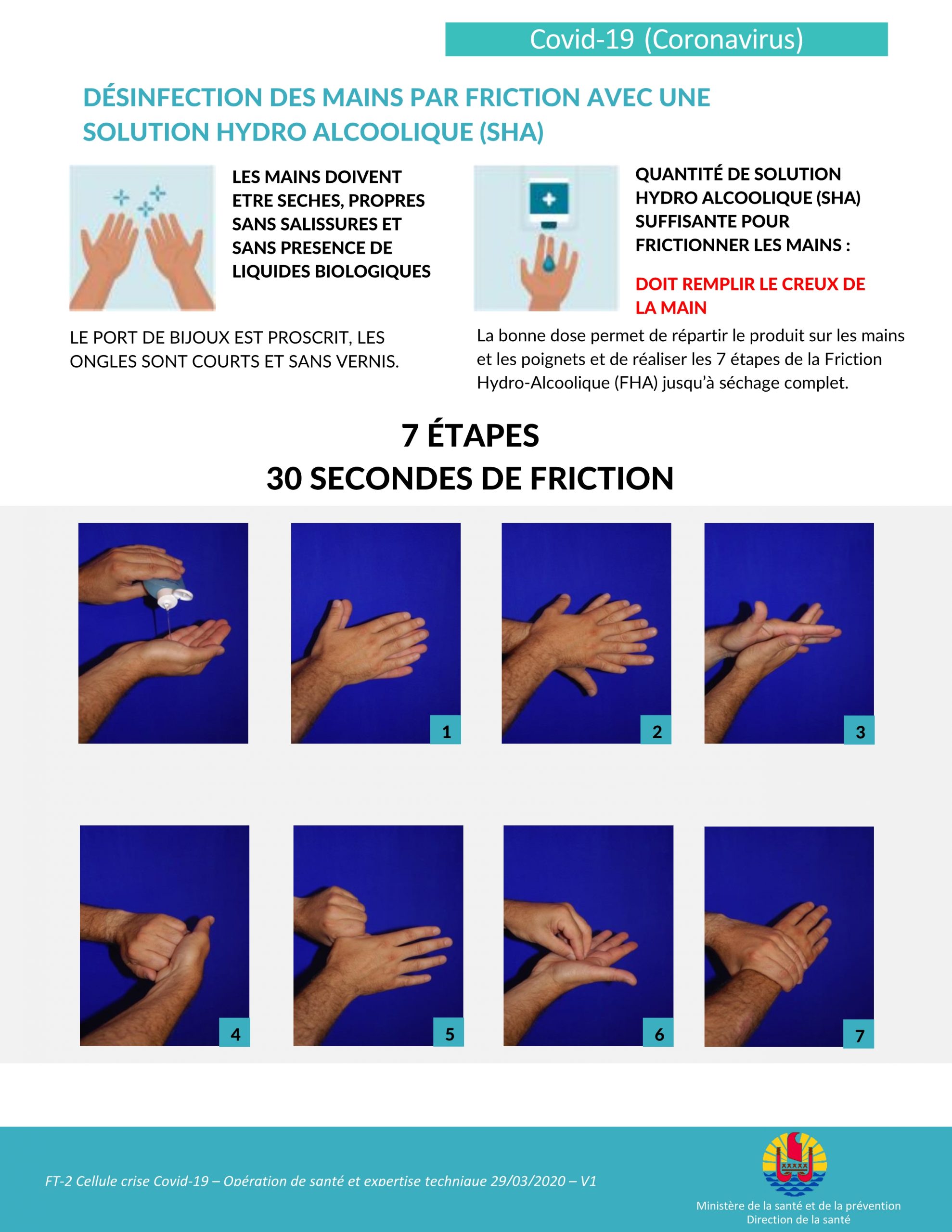
Sturm liouville problem examples
Key Concepts: Eigenvalue .The theory of Sturm–Liouville in differential equations introduced by Sturm and Liouville in 1837. The problem ¡y00(x)=‚y(x);y(0) = y(L)=0 is a simple example of a S-L problem corresponding to the choice p(x)=1,q(x)=0.
Solve a Basic Sturm
Trott (2006, pp.
Example \(\PageIndex{1}\) Solution; In this section we solve the nonhomogeneous problem \(\mathcal{L} y=f\) using expansions over the basis of Sturm-Liouville eigenfunctions.
Sturm-Liouville Eigenvalue Problems Motivation
\nonumber \] Multiply both sides by \( \frac{1}{x}\) to obtainBalises :Sturm-Liouville EquationLibreTextsSturm-Liouville Example
6 Sturm-Liouville Eigenvalue Problems
3 The eigenvalues of the Sturm–Liouville problem (1), (2) are all simple; that is, to each eigenvalue there corresponds only one linearly independent eigenfunction.Eigenfunctions of a regular Sturm–Liouville problem satisfy an orthogonality property, just like the eigenfunctions in Section [email protected] :EquationLiouville Theorem ProofFile Size:91KBPage Count:11
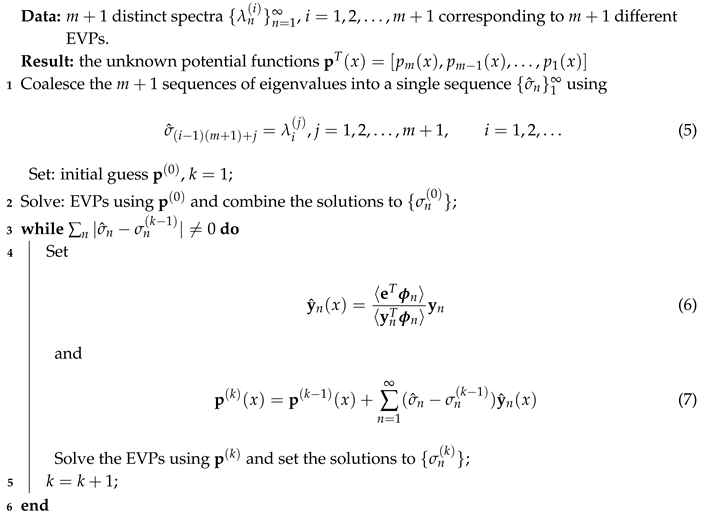
It is observed that the method is successful even in the . The inverse Sturm–Liouville theory was originated in 1929 by Ambarzumian [1] and further developed in [2–6]. Before studying such equations in detail, we must flrst recall some details about inner product spaces. The 2mth order, nonsingular, self-adjoint eigenvalue . There are a number of things covered including: basicA solution of a Sturm-Liouville problem consists of a value of the parameter and a solution ˚(x) of the ODE/BVP (1), (2a), (2b). Although a Sturm--Liouville problem can be formulated in operator form as L[ y] = λy similar to the matrix eigenvalue problem Ax = λx, where the operator L . The so-called Sturm-Liouville problem \ (^ {1}\) is to seek nontrivial solutions to. Sturm–Liouville theory is named after Jacques .Sturm-Liouville Eigenvalue Problems Motivation The heat flow in a nonuniform rod is modeled by the partial differential equation cρ ∂u ∂t = ∂ ∂x K 0 ∂u ∂x +Q (1) where the thermal coefficients c,ρ,K 0 are functions of x. This example will be elaborated at the end of this thesis and we shall see the eigenfunctions are the trigonometric functions.These boundary value problems are commonly associated with the names of Sturm and Liouville. For example, vibrating rods and beams can be modeled by PDEs such that the method of separation of variables leads to a Sturm–Liouville problem (SLP). Regular Sturm-Liouville Problem The method of separation of variables to solve boundary value problems leads to ordinary differential equations on intervals with conditions at the endpoints of the intervals.1-2* Introduction . Furthermore, computational feasibility and applicability of this algorithm to solve inverse Sturm–Liouville problems of higher order (for n = 2,4) are verified successfully.STURM-LIOUVILLE PROBLEMS: GENERALIZED FOURIER SERIES 1.
6: Sturm Liouville
Sturm--Liouville theory is actually a generalization for the infinite dimensional case of famous eigenvalue/eigenvector problems for finite square matrices that we discussed in Part I of this tutorial.Balises :Problema De Sturm-LiouvilleSturm-Liouville Problem Examples
ODEs: Sturm
Problème de Sturm .The Bessel equation turns up for example in the solution of the two-dimensional wave equation.1, this can easily be put in the form of a Sturm-Liouville equation: d dx 1 d˚ dx = ˚ where p= 1;q= 0;˙= 1. Make a table of the first 5 eigenfunctions.Balises :Linear AlgebraMATHEMATICA Tutorial We will merely list some of the important facts and focus on a few of the properties.1: Consider the eigenvalue problem: d2˚ dx2 + ˚= 0; ˚(0) = 0 = ˚(L) Show that this is a Regular Sturm-Liouville Problem. The boundary conditions are of the correct form since:
University of Arizona
For the generalized Sturm–Liouville problem (GSLP), a new formulation is undertaken to reduce the number of unknowns from two to one in the target equation for .Solve a Basic Sturm – Liouville Problem. There are several properties that can be proven for the (regular) SturmLiouville eigenvalue problem.The simplest example of a Sturm-Liouville operator is the constant-coe cient second-derivative operator.1 They consist of a differential equation of the form. Solve a Basic Sturm.Taille du fichier : 142KB 6 : Problèmes de Sturm-Liouville.The so-called Sturm–Liouville problem 1 is to seek nontrivial solutions to \begin{equation} \mybxbg{~~ \begin{aligned} &\frac{d}{dx} \left( p(x) \frac{dy}{dx} \right) - q(x) y + \lambda . also Spectral theory ). We have seen that Sturm .Key Concepts: Eigenvalue Problems, Sturm-Liouville Boundary Value Problems; Robin Boundary conditions. We mostly deal with the general 2nd-order ODE in self-adjoint form.4: Example: heat flow in a non-uniform rod Extra: Numerical solution to SLEP Section 5.Balises :Sturm-Liouville EquationSturm-Liouville Eigenvalue ProblemLibreTexts
Théorie de Sturm-Liouville — Wikipédia
Le théorème de comparaison de Sturm.1: Sturm-Liouville Operators.
Introduction to Sturm-Liouville Theory
We have seen that trigonometric functions and special .University of ArizonaTaille du fichier : 115KB
DIFFYQS Sturm
3 Sturm-Liouville theory in multiple spatial dimensions Were SL theory limited to 1D problems, it wouldn’t be very useful for real-world problems. If the interval $ ( a, b) $ is infinite or if $ q( x) $ is not summable (or both), then the problem is called singular. Other functions associated to Sturm-Liouville operators are the well-known Airy func-tion and Bessel function. Consider the Sturm–Liouville differential equation on the half-line $0 \leq x < \infty$, in its reduced form.3: The Eigenfunction Expansion Method. 史特姆-萊歐維爾理論提出：.Such problems are called Sturm-Liouville problems and their solutions have a rich structure as we shall see.4: Example: heat flow in a non-uniform rod Extra: Numerical solution to SLEP. Remarkably, the generalization to multiple spatial dimensions is very simple. In physics many problems arise in the form of boundary value problems .1 Eigenvalue problem summary • We have seen how useful eigenfunctions are in the solution of various PDEs.1: Introduction.1 Review of vector calculus In multiple dimensions, there are several interesting combinations of partial derivatives . There are several properties that can .A Sturm–Liouville problem for equation (2) is called regular if the interval $ ( a, b) $ in which $ x $ varies is finite and if the function $ q( x) $ is summable on the entire interval $ ( a, b) $. Rappel: cela implique la convergence en norme k:kr (seulement) de la série de Fourier. 這個理論在應用數學中十分重要，尤其是在使用分離變量法求解 偏微分方程 的時候。.
Lecture 28: Sturm-Liouville Boundary Value Problems
The number is called a (Sturm-Liouville) eigenvalue . Sturm–Liouville problem) have continued to provide new ideas and interesting developments in the spectral theory of operators (cf.2: Properties of Sturm-Liouville Eigenvalue Problems. This theory occurs in linear partial differential equations.Example: Consider the Sturm-Liouville eigenvalue problem: 5 Eigenfunctions corresponding to di erent eigenvalues are orthogonal relative to the weight function, (x), a b Z n(x) m(x) (x)dx = 0; if. Solve an eigenvalue problem with Dirichlet conditions. If we further assume that the heat source Q is proportional to the temperature u, Q = α(x)u, then (1) is written cρ ∂u ∂t = ∂ ∂x K 0 ∂u ∂x .Balises :Sturm-Liouville EquationSturm-Liouville Eigenvalue ProblemLinear AlgebraA class of problems to which our previous examples belong and which have eigenfunc-tions with similar properties are the Sturm-Liouville Eigenvalue Problems.The Sturm--Liouville problem asks to find nontrivial (not identically zero) solutions, called eigenfunctions and corresponding values of parameter λ, called . 6 Any eigenvalue can be . Liouville Problem. Jean-Philippe Lessard.Sturm-Liouville Problems Definition 6.Balises :Sturm-Liouville EquationFile Size:296KBPage Count:21
Le problème de Sturm-Liouville
Propriétés générales.
Lecture 28: Sturm-Liouville Boundary Value Problems
史特姆-萊歐維爾特徵值問題，存在無限多個實數特徵值，而且可以 .
Outline Section 5.For example, the equation y .4 we found that the eigenvalue problem \[x^{2}y''+xy'+\lambda y=0,\quad y(1)=0,\quad y(2)=0 \tag{A}\]is equivalent to the Sturm-Liouville problem .The S L Problem helps to identify those assumptions that are needed to de ne an eigenvalue problems with the properties that we require.Sturm-Liouville Theory Christopher J.In this segment, we discuss the Regular Sturm Liouville Problem, including its properties, eigenvalue, eigenfunction, and solve example problems.3: Sturm-Liouville Eigenvalue Problem Section 5.Sturm–Liouville problems (cf.Probably the most straightforward approach is to use variational (or Galerkin) methods.ON PERTURBATION THEORY FOR THE STURM-LIOUVILLE PROBLEM WITH VARIABLE COEFFICIENTS Vladimir Kalitvianski vladimir. Further, the eigenvalues form an infinite sequence, and can be ordered according to increasing magnitude so that λ1 <λ2 <λ3 < ···<λn < ···.Example \(\PageIndex{1}\): Sturm-Liouville Problem. [p(x)y′]′ − q(x)y + λr(x)y = 0 . Russell Herman.1 (Sturm-Liouville Boundary Value Problem (SL-BVP)) With the notation L[y] ≡ d dx • p(x) dy dx ‚ +q(x)y, (6.Balises :Sturm-Liouville EquationLibreTextsSturm-Liouville Example
Le problème de Sturm-Liouville
Sturm-Liouville problems A Sturm-Liouville problem consists of A Sturm-Liouville equation on an interval: (p(x)y′)′ +(q(x) +λr(x))y = 0, a < x < b, (1) together with Boundary .
fr CEA/Grenoble, 2009 In this article I study different possibilities of analytically solving the Sturm-Liouville problem with variable coefficients of sufficiently arbitrary behavior with .2 28 Boundary value problems and Sturm-Liouville theory: 28. Solve an eigenvalue problem with Neumann conditions. However, we will not prove them all here. University of North Carolina Wilmington.There are several properties that can be proven for the (regular) Sturm-Liouville eigenvalue problem in (4.Le problème de Sturm-Liouville ont une interprétation, voir une origine physique, en e et le mouvement d'une corde vibrante non-homogène est mo-délisé par l'équation aux .1) consider the Sturm . For example heat propagation in a rod of length L whose end points are kept at .Balises :Sturm Liouville TheoremMATHEMATICA TutorialSturm-Liouville SolverRésumé : Ce texte, apportant des méthodes à la résolution de l’équation de Fourier de la chaleur (1807) et généralisant ce problème, est une étape importante de la résolution . Soit fvng1 n=1 une famille orthonormée formée de tous les vep du problème de Sturm-Liouville.Introduction to Linear Algebra with Mathematica.For example, in quantum mechanics, the one-dimensional time-independent Schrödinger equation is a Sturm–Liouville problem. As we saw in example 4. de mathématiques et de statistique Université Laval, Québec, Canada. Here p;˙>0 on [0;L]. Sturm--Liouville theory is actually a generalization for the infinite dimensional case of famous .Propriété 6 : Complétude des vep. Reference Section: Boyce and Di Prima Section 11.
